Study of Rheological Behavior of Olive Oil Used as Biodegradable Lubricant

Introduction

Olive oil can be obtained by one of the following methods: chemical extraction involves treating the olives with chemicals (various organic solvents) that help separate the oil from the other components of the olives.

Mechanical extraction by hot pressing in which the olives are pressed to squeeze the oil from them. It is called “hot pressing” because during this process the heat is released and the olive paste is heated.

Mechanical extraction by cold pressing, which unlike hot pressing, this process involves pressing the olives and squeezing the oil from them in a controlled environment, which ensures a low temperature. This method is also the one through which the oil keeps most of its nutrients.

Furthermore, the process of obtaining olive oil may involve refining. By refining, however, a significant amount of nutrients is lost from the oil. Refining involves treating the oil – with chemicals, exposure to very high temperatures – the purpose of this process being to remove impurities. Refining olive oil increases its shelf life, but also fades its flavors. However, you can also find raw, unrefined olive oil, with an intense olive aroma and a higher acidity.

Both virgin and extra virgin olive oil are obtained by cold mechanical methods, so without the use of chemical solvents. Both types have the same level of antioxidants and nutrients, but differ in oleic acid concentration. At a concentration of up to 0.8% oleic acid, the oil is considered extra virgin, and between 0.8 and 2% is considered virgin.

As you saw above, olive oil can be of several kinds and, depending on how it is produced, it can provide you with a different level of nutrients. No matter what type of olive oil you choose, it is certain that you will have most of the benefits of this product. It is ideal to have in the house both a refined olive oil, which you can use for cooking, and a virgin or extra virgin olive oil for salads, soups, cold sauces, etc.

Problems choosing olive oil arise when we talk about counterfeit products, which contain chemicals meant to mimic the color, taste and density of olive oil, but which have a much lower price. Here are some ways you can make sure the olive oil you plan to buy is truly quality ^1-14.

Andrade equation showing the dependence of dynamic viscosity versus temperature ^{14, 15, 16, 17, 19}:

By logarithm equation (1) we obtain

The last equation is used for making linear the equation (1).

Esteban et al. [16] recommend the Azian equation (3) [18,19], they introduced another equation with three constants:

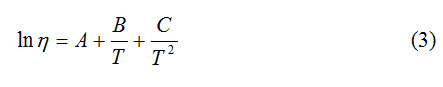
where T is the absolute temperature and A, B and C are constant of the material.

In this article we found three relationships of viscosity dependence temperature by fitting experimental data. The polynomial and exponential relations found starting from the Andrade equation accurately describe the non-Newtonian behavior of olive oil.

Material and methods

Olive oil is a liquid fat obtained from olive produced by pressing whole olives and extracting the oil. Olive oil was obtained by hot pressing. At a concentration of up to 0.8% oleic acid, the oil is considered extra virgin. Extra virgin olive oil produced in Romania was studied with the Haake VT 550 viscometer equipped with a VH₁ sensor for shear speeds between 3 and 120s^-1 and viscosities dynamic between 10⁴ and 10⁶ mPa.s. The determinations were made at temperatures between 40 and 100 from 10 to 10⁰C.

The physico-chemical characteristics of the olive oil are: refraction index 1.4677– 1.4705; the mass fraction of water and volatile substances 0.2%, the mass fraction of the impurities other than those from the fats (mass sediment) 0.1%, saponification index = 184- 196 mg KOH/g, iodine index = 75-94 g I2/100g, mass fraction of non-saponifiable substances max 15%, relative density at 20ºC = 0.910 – 0.916; 20 mmol oxigen activ/kg.

Result and discussion

Rheograms 1, 2 and 3 of olive oil at shear rates of 6 s^-1, 17.87 s^-1 and 52.95 s^-1. There is a decrease in dynamic viscosity with temperature and shear rate at high temperatures due to the orientation of the oil molecules.

Figure 1: Rheogram of olive oil at shear rate of 6 s-1 Click here to View figure

Figure 2: Rheolgram of olive oil at shear rate of 17.87 s-1 Click here to View figure

Figure 3: Rheogram of olive oil at shear rate of 52.95 s-1 Click here to View figure

The decrease in dynamic viscosity is more pronounced in the temperature range 40-60⁰C degrees Celsius compared to the temperature range 60-100⁰C. At the shear rate of 52.95 s^-1 the decrease is 63%, in the temperature range of 30ºC – 60ºC. For the temperature range of 60ºC – 100ºC, the decrease is 44.4%.

Equations (4) – (6) were obtained by polynomial and exponential fitting of the experimental data of olive oil. ²⁰

The parameters of equations A, B, C and D as well as the correlation coefficients are included in tables 1,2, and 3.

The correlation coefficients have values between 0.8754 and 0.9999.

where T is the absolute temperature and A, B, C and D are constant of the material.

Table 1: Parameters equation (4) and correlation coefficient R² [20]

Shear rate, s-1	Correlation parameters (eq. 4)			Correlation coefficient, R2
	A	B	C
3.30	1077.6917	-5.9273	0.0083	0.9475
6.00	941.3095	-5.2266	0.0074	0.8942
10.60	739.5499	-4.0639	0.0057	0.9177
17.87	628.4163	-3.4335	0.0048	0.9045
30.00	682.6112	-3.7636	0.0053	0.8772
52.95	705.4780	-3.8999	0.0055	0.9077
80.00	702.5944	-3.8844	0.0054	0.8754
120.0	208.7929	-1.0777	0.0014	0.9886

Table 2: Parameters equation (5) and correlation coefficient R² [20]

Shear rate, s-1	Correlation parameters (eq.5)				R2
	A	B	C	D
3.30	9947.2339	-83.8129	0.2358	-2.21111E-4	0.9874
6.00	11337.3934	-96.517	0.2740	-2.59167E-4	0.9842
10.60	8427.9721	-71.5778	0.2029	-1.91667E-4	0.9793
17.87	8428.2649	-71.9260	0.2049	-1.94444E-4	0.9807
30.00	9429.5843	-80.5796	0.2296	-2.18056E-4	0.9740
52.95	8705.8941	-74.1537	0.2107	-1.99444E-4	0.9886
80.00	9460.7101	-80.7917	0.2300	-2.18333E-4	0.9685
120.0	1230.8024	-9.9127	0.0269	-2.43519E-5	0.9925

Table 3: Parameters equation (6) and correlation coefficient R² [20]

Shear rate, s-1	Correlation parameters (eq.6)			R2
	η0	A	B
3.30	15.4796	7.42971E15	9.3010	0.9868
6.00	12.2465	1.50282E13	11.3020	0.9842
10.60	10.6018	9.44701E11	12.5041	0.9859
17.87	9.4467	1.11163E10	15.1372	0.9696
30.00	9.0584	1.19194E11	13.5677	0.9617
52.95	8.3325	1.96634E11	13.2852	0.9809
80.00	8.1306	2.28666E12	12.0368	0.9703
120.0	7.9697	1.9415E13	12.0548	0.9658

 

Conclusion

The article proposes three relations obtained by exponential and polynomial fitting of the experimental data starting from the Andrade equation. The relationships found accurately describe the curves obtained so the correlation coefficients are close to unity.  The correlation coefficients are close to unity which shows that the three equations found accurately describe the behavior of olive oil.

Acknowledgement

None

Conflict of interest

None

References

1. Crudu I., 2008, Tribosistem-Tribomodel în studiul sistemelor mecanice, Galaţi University Press, Galaţi.
2. Hohn B.R., Michaelis K., Dobereiner R., 1999, Lubr. Eng., p. 15.
3. Aguilera M.P., Beltran G., Ortega D., Fernandez A., Jimenez A., Uceda M., 2005, Food Chemistry, 89, 387-391.
   CrossRef
4. Gouveia J.M.B., 1997, OLIVAE, 66, 34-45.
   CrossRef
5. Dais P., Hatzakis E., 2013, Analytica Chimica Acta, 765, 1-27.
   CrossRef
6. Lazzez A., Perri E., Caravita M.A., Khlif M., Cossentini M., 2008, Journal of Agricultural and Food Chemistry, 56, 982-988.
   CrossRef
7. Kandylis P., Vekiari A.S., Kanellaki N., Kamoun Grati N., Msallem M., Kourkoutas Z., 2011, Food Science and Tehnology, 44, 1333-1341.
   CrossRef
8. Vekiari S.A., Papadopoulou P., Kiritsakis A., 2007, Grasas Y Aceites, 58, 237-242.
   CrossRef
9. Stanciu I.,  2011, Journal of Science and Arts, 1, 55-58.
10. Goodrum J.W., Law S.E., 1982, Am. Soc. Agic. Eng, 897-900.
    CrossRef
11. Mustafa E.T., Gerpen J.H.V., 1999,  J. Am. Oil Chem. Soc., 76, 1511-1513.
    CrossRef
12. Kosmert T., Abramovic H., Klofutar C., 2000,  J. Food Eng., 46, 165-171.
    CrossRef
13. Wan Nik W., Ani F.N., Masjuki H.H., Eng Giap S.G., 2005, Industrial Crops and Products, 22, 249-255.
    CrossRef
14. Rodenbush C.M., Hsieh F.H., Viswanath D.S., 1999, J. Am. Oil Chem. Soc., 76-141.
    CrossRef
15. Krisnangkura K., Yimsuwan T., Pairintra R., 2006, Fuel, 85-107.
    CrossRef
16. Esteban B., Riba J.R., Baquero G., Rius A., Puig R., 2012, Biomass and Bioenergy, 42, 164-171.
17. Stanciu I., 2013, Journal of Science and Arts, 4(25), 355-360.
    CrossRef
18. Azian M.N., Kamal A.A.M., Panau F., Ten W.K., 2001, J. Am. Oil Chem. Soc., 78, 1001.
19. Solea L.V., Deleanu L., Georgescu C., 2013, Mechanical Testing and Diagnosis, 3, 44-48
20. Stanciu I., 2012,  Ovidius University Annals of Chemistry, 23(1), 27-30.
    CrossRef

---

This work is licensed under a Creative Commons Attribution 4.0 International License.