Time Domain Reflectometric Studies on Methylcellosolve – Acetylacetone Binary Solutions

Introduction

2-Methoxyethanol, also known as Methylcellosolve (MCS), is an organic compound which has H – bond donor as well as acceptor groups ¹, the O -H, CH₃  and CH₂  are the donor groups and the C – O – C is the hydrogen bond acceptor group. It can be used as a solvent for electrolytes and has many industrial applications ^{2 – 4} . It is essential to know the interacting properties of MCS through intermolecular H – bonds, which are very important type of non-covalent interaction forces that operate among homo as well as hetero molecules and play an important role in determining the shape, structure and properties of molecules ^{5 – 7}, with other molecules in fundamental research as well as industrial applications point of view. The interacting behaviour of MCS in the presence of various polar molecules has been studied by many researchers ^{8 – 11} using the techniques such as ab initio calculations, Raman spectroscopy, ultrasonic studies, FTIR studies etc. Each technique is capable of providing its own characteristic information regarding the molecular interactions.  Dielectric spectroscopy is an effective tool that can be applied to obtain unique information regarding the fluid structure and has been employed to study the associating behaviour of MCS with chlorobenzene ¹², a substituted benzene, and ethyl acetate ¹³ by our research group. In the present work MCS is dissolved in acetylacetone (ACACT), which belongs to the diketone class of compounds that exist as a mixture of keto and enol tautomers and is used as a chelating reagent for its ability of involving in coordinate complexation with metals ¹⁴, at various mole fractions and the associating behaviour of MCS in the solutions have been investigated with the help of the parameters obtained using dielectric spectroscopy.

Materials and methods

Acetylacetone and methylcellosolve (anhydrous 99.8%) were purchased from Sigma Aldrich Pvt. Ltd, USA and are used as received.The time-domain reflectometric studies on pure ACACT, MCS, and their binary solutions in the concentration range 0 ≤ X ≤ 1 of was carried out with the help of a Tektronix Digital Serial Analyzer DSA 8200 sampling mainframes with the sampling module 80E08 in the frequency range:  10 MHz – 32 GHz at 25^oC ^{15, 16}. The complex permittivity spectra ε*  (ω) have been obtained using the method of non-linear least square fit ^{17, 18}. Mass measurements have been made using a digital analytical balance supplied by Eagle Scale Manufacturing Works, Gujarat was used.

Theory

The complex dielectric permittivity spectra can be fitted using the Havriliak-Negami (HN) function ¹⁹

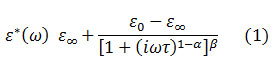
where α (0 ≤ α ≤ 1) and β (0 ≤ β ≤ 1) are the shape parameters, ω is the angular frequency, τ is the relaxation time, ε₀ is the static dielectric constant and ε₀₀ is the dielectric constant at high frequency. The Debye, Cole-Cole and Cole-Davidson relaxation models are described by (α = 0, β = 1), (0 < α ≤ 1, β = 1) and (α = 0, 0 < β ≤ 1), respectively.

The alignment of dipoles in a pure polar liquid can be obtained from the Kirkwood correlation factor g ²⁰

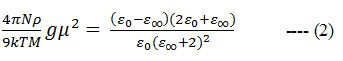
where μ, ρ, M and k are the gas phase dipole moment, density, molecular weight and the Boltzmann constant, respectively.

The dipolar alignment in a polar – polar binary liquid mixture is given by the effective Kirkwood correlation factor ( g^eff) ²¹

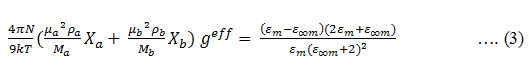
Here N and X are the Avogadro number and the mole fraction, respectively. a, b and m represent the liquid a, liquid b and the mixture, respectively.  The corrective Kirk wood correlation factor g _f ²² is calculated using the relation

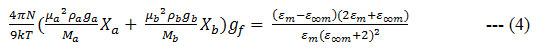
The excess permittivity is a parameter which can be used to investigate the heteromolecular interactions a solution has been calculated using the following four in formulae ^{22 – 25}

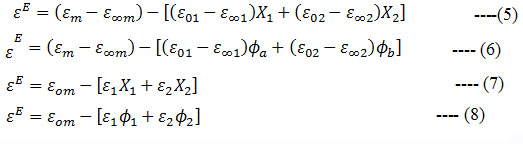
where ϕ is the volume fraction. The excess permittivity values have been fitted using the Redlich-Kister equation ^{26, 27}

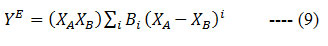
with B_i as the Redlich-Kister coefficients.

Result and discussions

The variation of dielectric permittivity (ε’ ) and dielectric loss (ε” ) with the logarithmic frequency has been presented in Fig. 1.The Cole- Davison model has been used for all the solutions (0 ≤ X2 ≤ 0.9) except pure ACACT which was fitted using the Debye model. The static permittivity ε_m and relaxation time τ values for pure MCS and ACACT in the present work are found to be close to the literature values[28, 29]. The relaxation time (τ) of neat ACACT is 14.02 ps and that of neatMCS is 29.72 ps (Table 1)which is greater than τ value of ACACT. This higher relaxation time of MCS is due to the formation of multimers. Yaseen et al. [30] reported that the co-operative process of multimers O – H ⋯O linkage give rise to high relaxation time. So, the relaxation time can be used to investigate the size of the rotating molecular unit and not for identifying the specific H – bond interaction sites of the solution constituents. The value of τ slowly decreases towards the value of pure ACACT (Table 1) when its mole fraction is increased from 0 to 1. The rate at which the τ value decreases is more in MCS rich solutions when compared with that in ACACT rich solutions. This type of changes in τ value signifies that the size of the MCS multimer units got affected much in MCS rich solutions and this in turn means that the formation of heteromolecular H – bonds is more favoured in MCS rich solutions.

Figure 1: The graph of a) dielectric permittivity (ε’) and b) dielectric loss (ε”) against LOG F for MCSAcac solutions at 298 K. Click here to View figure

Table 1: Density (ρ), Static permittivity (ε_om), permittivity at high-frequency limit (ε_o∞)and relaxationz time  (τ), effective Kirk wood correlation factor (g^eff)and corrective Kirkwood correlation factor(g _f ) for the binary mixture of MCS with Acac. Here, X₂ / ϕ₂ is the mole/volume fractions of Acac at 298 K

X2	ϕ2	ρ (g/cm3)	εom	εo∞	τ (ps)	geff	g f
0.00	0.00	0.934	17.11	1.97	29.7	1.45	1.00
0.10	0.13	0.936	17.40	1.98	23.4	1.47	0.97
0.18	0.22	0.874	17.89	2.08	20.5	1.42	0.92
0.28	0.34	0.871	18.78	2.14	19.2	1.44	0.91
0.40	0.46	0.878	19.87	2.14	19.2	1.52	0.93
0.51	0.57	0.872	21.34	2.23	16.6	1.56	0.95
0.63	0.69	0.888	23.09	2.28	15.4	1.65	1.04
0.71	0.76	0.883	24.97	2.29	15.3	1.76	1.04
0.83	0.86	0.866	25.56	2.30	15.3	1.79	1.02
0.87	0.90	0.915	25.71	2.31	14.2	1.79	1.00
1.00	1.00	0.926	26.49	2.33	14.0	1.82	1.00

Figure 2: The dependency of the dielectric constant (εom) of the binary solutions on the mole fraction of Acac at 298 K. Click here to View figure

Non-linear variation of ε_om with the mole fraction of Acac can be noticed (Fig. 2) in the 0 ≤ X₂ ≤ 0.71  concentration range and linear change occurs in the remaining range of the concentration. The non-linearity of the ε_om vs X₂ has been reported in terms of homo as well as heterointeractions in various articles [31-33]. In the present work, the non-linear change has been attributed to the presence of H – bond interactions of MCS with Acacwhile the linear variation is due to the relatively weaker hetero interactions. The 𝑔^𝑒𝑓𝑓and g _f values calculated using mole and volume fractions remain the same (Table 1). The 𝑔^𝑒𝑓𝑓values of neat MCS and neat Acac are 1.45 and 1.82, respectively, which are the indicators that the dipoles of both MCS and Acac have parallel angular correlation. This parallel alignment of the dipoles continues to exist over the entire concentration range where the 𝑔^𝑒𝑓𝑓values are greater than one. But the parallel angular correlation has been reported ^{12, 13} only in MCS rich solutions when it is mixed with chlorobenzene (dipole moment = 1.69 D)/ethyl acetate (dipole moment = 1.78 D) which are relatively less polar when compared with the Acac (dipole moment = 2.78 D). The value of g _f , the measure of the degree of hetero interaction, is found to slightly deviates from unity indicating the presence of weak heteromolecular H – bond interaction forces in the binary solutions. The g _f  shows a bit more deviation in MCS + chlorobenzene/ethyl acetate ^{12, 13} binary solutions.

The excess dielectric permittivity ε^E values (Figs. 2 and 3) of the binary solutions computed using eqs. (5) – (8) qualitatively give the same information on the heteromolecular interactions with the exception of the point at which the transition from negative to positive regime takes place. Therefore, usage of mole fraction instead of volume fraction qualitatively causes no difference in the information regarding the heteromolecular interactions that are present in the binary solutions at different concentrations. According to most of the literature reports ^{23, 25, 34 – 37}, the positive deviation of ε^E  from ideal values is due to the formation of H – bonded structures with increased number of parallel aligned dipoles and the negative deviation is the result of the presence of heteromolecular interactions with a decrease in the number of parallel oriented dipoles. But our earlier research work ³⁸ has confirmed that the interpretation of positive/negative ε^E  values as the sign of increase/decrease of the number of dipoles is incorrect if the binary solutions consist of associative molecules. Therefore, the negative ε^E  values for MCS rich solutions can be taken as the sign of i) formation of heteroassociated molecular networks with smaller dipole moments and ii) the decrease in the degree of alignment of dipoles ^{39, 40}, that can be inferred from the relatively smaller 𝑔^𝑒𝑓𝑓values in MCS rich solutions, while the positive deviation of ε^E  values is the indication that heteromolecular complex has been formed with higher dipole moment ⁴¹.

Figure 3: Plot of a)  Excess permittivity εE=(εom-ε∞m) -[(ε1 – ε∞1) X1+((ε2-ε∞2) X2]against the mole fraction  of Acac and b) excess permittivity εE=(εom-ε∞m) -[(ε1 – ε∞1) ϕ1+((ε2-ε∞2) ϕ2] against the volume fraction  of Acac of MCSAcac binary solutions at 298 K. Click here to View figure

Figure 4: Plot of a)  Excess permittivity εE = εom-[ε1 X1 + ε2 X2] against the mole fraction of Acac b) excess permittivity εE = εom-[ε1 ϕ1 + ε2 ϕ2]  against the volume fraction (ϕ2) of Acac in  MCSAcac binary solutions at 298 K. Click here to View figure

Conclusions

Dielectric studies has been applied on the binary solutions of MCS with ACACT at different mole fractions of MCS/ACACT. The relaxation time of MCS slowly decreases towards the value of pure ACACT with the increase in its concentration. The rate of decrease in the τ value is found to be more in MCS rich solutions than in ACACT rich solutions and so the formation of heteromolecular H – bonds is more favoured in MCS rich solutions. The 𝑔^𝑒𝑓𝑓 values suggest that the dipoles of the heteroassociated molecular networks have parallel angular correlation. The excess dielectric permittivity ε^E  values have been calculated using the four formulae available in literature. All the four have qualitatively yield the same trend of deviation of  ε^E  values from the ideal values.

Acknowledgement

The authors thank Dr. A.C. Kumbharkhane,  School of Physical Sciences, S.R.T.M. University, Nanded – 431606 , Maharashtra, India for providing instrumentation facility. 

Conflict of interest

The authors declare that we have no conflict of interest.

References

1. Sengwa,R. J.; Madhvi, Sankhla, S.; Sharma, S.; Bull. Korean Chem. Soc.2006, 27,    718.
2. Srinivasa Krishna,T.; Narendra,K.; Gowrisankar,M.; Nain,A. K.; Munibhadrayya,B.; J. Mol. Liq.2017, 227, 333.
3. Piekarski,H.; Tkaczyk,M.; Thermochim. Acta1987, 122, 377.
   CrossRef
4. Piekarski,H.; Tkaczyk,M.; J. Chem. Soc. Faraday Trans.1995, 91, 2299.
   CrossRef
5. Hobza,P.; Havlas,Z.; Chem. Rev.2000, 100, 4253.
   CrossRef
6. Scheiner,S.; Hydrogen Bonding; Oxford University Press: New York, 1997.
7. Hobza,P.; Sponer,J.; J. Chem. Rev.1999, 99, 3247.
   CrossRef
8. Amorim da Costa,A.M.; Duarte,A.S.R.; Amado,A.M.; Vib. Spectrosc.2006, 42, 302.
   CrossRef
9. Satyanarayana,G.R.; Bala Karuna Kumar,D.; Sujatha,K.; Lakshmanarao,G.; Rambabu,C.; J. Mol. Liq. 2016, 216, 526.
   CrossRef
10. Balaji,R.; Gowri Sankar,M.; Chandra Sekhar,M.; Chandra Shekar,M.; J. Mol. Liq.2016, 216, 330.
    CrossRef
11. Mohan Krishnan,K.; Ramababu,K.; Ramachandran, D.; Venkateswarlu,P.; Raman,G.K.; Fluid Phase Equilib.1995, 105, 109.
12. Mahendraprabu,A.; Karthick,N.K.; Kannan,P.P.; Sangeetha,T.; Kumbharkhane,A.C.;  Arivazhagan,G.; J. Mol. Struct.2020, 1205, 127565.
    CrossRef
13. Mahendraprabu, A.; Sangeetha, T.; Kannan, P.P.; Karthick, N.K.; Kumbharkhane, A.C.; Arivazhagan, G.; J. Mol. Liq.2020, 301, 112490.
    CrossRef
14. El-Hendawy,A.M.; J. Mol. Struc.2011, 995, 97.
    CrossRef
15. Kumbharkhane,A.C.; Joshi,Y.S.; Mehrotra,S.C.; Yagihara,S.; Sudo, S.; J. Physica B, 2013, 421, 1.
16. Rander,D.N.; Joshi,Y.S.; Kanse,K.S.; Kumbharkhane,A.C.; J. Mol. Liq.2014, 199, 367.
    CrossRef
17. Cole,R.H.; Berberian,J. G.; Mashimo,S.; Chryssikos,G.; Burns,A.; Tombari,E.; J. Appl. Phys.1989, 66, 793.
    CrossRef
18. Kumbharkhane,A.C.; Puranik,S.M.; Mehrotra,S.C.; J. Chem. Soc. Faraday Trans.1991, 87, 1569.
19. Havriliak,S.; Negami,S.; J. Poly. Sci. Part C1966, 14, 99.
    CrossRef
20. Frolhich,H.; Theory of dielectrics, Oxford University Press, London, 1949.
21. Puranik,S.M.; Kumbharkhane,A.C.; Mehrotra,S.C.; J. Mol. Liq.1991, 50, 143.
    CrossRef
22. Hasted,J.B.; Aqueous Dielectrics, Chapman and Hall, London, 1973.
23. Ghanadzadeh Gilani, A.; Ghanadzadeh Gilani,H.; Ansari,M.; Ojani,N.; J. Chem. Thermodyn. 2012, 44, 44.
24. Mahendraprabu,A.; Kumbharkhane,A. C.; Joshi,Y. S.; Shaikh,S. S.; Kannan,P. P.; Karthick,N. K.; Arivazhagan,G.; J. Mol. Struct.2017, 1136, 303.
    CrossRef
25. Alonso,V.; González,J. A.; García de la Fuente,I.; Cobos,J. C.; Thermochim. Acta2012, 543, 246.
26. Al-Azzawal,S.F.; Awwad,A.M.; Al- Dulaiji,A.M.; Al-Noori,M.K.; J. Chem. Eng.  Data1990, 35, 463.
27. Aralaguppi,M.I.; Aminabhavi,T.M.; Balundgi,R.H.; Joshi, Y.S.; J. Phys. Chem.1991, 95, 5299.
    CrossRef
28. Mahendraprabhu,A.; Sangeetha, T.; Kannan,P.P.; Karthick,N.K.; Kumbharkhane, A.C.; Arivazhagan,G.; J. Mol. Liq.2020, 301,
    CrossRef
29. David R. LIDE CRC Handbook Of Chemistry And Physics 85^th Edition , 2005.
30. Yaseen,S.A.; Under,P.B.; Saif,F.A.; Patil,S.S.; Khirade,P.W.; Ferroelectrics2017, 519, 49.
31. Gilani, A.G.; Gilani, H.G.; Ansari, M.; J. Chem. Thermodyn.2012, 55, 203.
    CrossRef
32. Gilani, A.G.; Ghanadzadeh, H.; Bahrpaima, K.; Ranjkesh, A.; J. Chem. Thermodyn.2010, 42, 967.
    CrossRef
33. Gilani, A.G.; Gilani, H.G.; Ansari, M.; Ojani, N.; J. Chem. Thermodyn.2012, 44, 44.
34. Shinde,M.; Talware,R.; Hudge,P.; Joshi,Y. S.; Kumbharkhane,A. C.; Pramana J. Phys. 2012, 78, 297.
35. Joshi,Y.S.; Hudge,P.G.; Kumbharkhane,A.C.; Mehrotra,S.C.; J. Mol. Liq.2011, 163, 70.
    CrossRef
36. Ghanadzadeh Gilani,A.; Ghanadzadeh,H.; Bahrpaima,Kh.; Ranjkesh,A.; J. Chem. Thermodyn. 2010, 42, 967.
37. Sengwa,R.J.; Choudhary,S.; Dhatarwal,P.; J. Mol. Liq.2018, 252, 339.
    CrossRef
38. Arivazhagan, G.; Shanmugam, R.; Elangovan,A.; Spectrochim.Acta Part A 2013, 105, 102.
    CrossRef
39. Arivazhagan,G.; Elangovan,A.; Shanmugam,R.; Vijayalakshmi,R.; Kannan,P.P.; Chem. Phys. Lett.2015, 627, 101.
40. Lata,S.; Singh,K.C.; Ahlawat,S.; J. Mol. Liq.2009, 147, 191.
    CrossRef
41. Arivazhagan,G.; Shanmugam,R.; Thenappan,T.; J. Mol. Struct. 2011, 990, 276.
    CrossRef

---

This work is licensed under a Creative Commons Attribution 4.0 International License.