Theoretical Approach of the Adsorption of Herbicide Amitrole on the Soil using DFT Method

Introduction                                                                     

Amino-1, 2, 4-triazole (ATA) is an active substance of pesticides. Potentially dangerous when applied to plants, this compound must be controlled to provide quality in food and in ecosystem. Indeed, it diminishes its capacity to capture the light and thus slows its growth.^1-4 Previous experimental results suggested the formation of hydrogen bonds between the herbicide and humic acid.^5-6 It appears that these bonds are responsible of adsorption of soluble humic substances and thus transport of amitrole in underground layers.

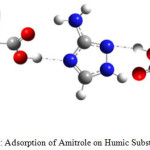Figure 1: Adsorption of Amitrole on Humic Substances Click here to View figure

 

The main objective of this paper is the study of electronic structures, properties and reactivity of this herbicide with humic substances (Figure 1) that have heterogeneous structure with hydrophilic and hydrophobes areas.^7-9 Frontier molecular orbital and chemical characteristics in aqueous solutionhave been used to explain the adsorption of herbicide in soil. The reactivity is related to global and local reactivity indices.¹⁰ The chemical characteristics in aqueous solution have been determined by Fukui indices approach. The results obtained predict that the studied compounds have pseudo-aromatic structure explaining interaction between bonds, large dipole moment and significant ability to produce a hydrogen bond.

Materials and Methods

Theoretical calculations are based on the Density Functional Theory¹¹ using the hybrid functional B3LYPwith 6-311G++(d,p) basis set through Gaussian09 software.¹² Graphical interface Gauss View package¹³ is used for building and viewing the results. The electronic structures of the stationary points were determined using Becke’s three-parameter hybrid exchange functional associated with the gradient-corrected correlation functional of Lee, Yang, and Parr B3LYP methods¹⁴ and have been analyzed by the natural bond orbital method (NBO).^15-17 Following energy minimization, frequency calculations were made in order to verify that all harmonic vibration frequencies have real values indicating that the structure represents a local minimum of energy. To take account of solvation effects, polarisable continuum model (PCM) was used.¹⁸

Results and Discussion

Structural and Energy Study

The results obtained for the optimized structures of 3-aminotriazole 3(ATA) and 5-aminotriazole 5(ATA) are reported in Figure 2, Table 1 where values in parentheses are determined for aqueous solution.

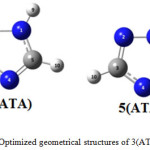Figure 2: Optimized geometrical structures of 3(ATA) and 5(ATA). Click here to View figure

 

Table 1: Calculated structural parameters of 3(ATA): bond distances in Å and angles in°

B3LYP	3(ATA)
6- 311G++(d,p)
N1-N2	1.368 (1.369)	N2N1 H9	119.92 (120.59)
N2-C3	1.326 (1.328)	C5N1H9	129.83 (129.07)
C3-N4	1.368 (1.367)	N2C3N6	123.09 (123.16)
N4-C5	1.319 (1.326)	N1C5H10	123.63 (123.45)
C5-N1	1.344 (1.337)	N4C5H10	126.20(126.42)
C3-N6	1.379 (1.381)	C3N6H7	114.12 (113.76)
C1-H9	1.007 (1.009)	C3N6H8	114.96 (114.48)
C5-H10	1.079 (1.079)	H7N6H8	114.16 (112.32)
N6-H7	1.009 (1.011)	N1N2C3N4	0.34 (0.18)
N6-H8	1.009 (1.011)	N2C3N4C5	-0.29 (-0.07)
N1N2C3	101.67 (101.89)	C3N4C5N1	0.10 (-0.09)
N2C3 N4	115.04 (114.69)	N1N2C3N6	-176.99 (-176.64)
C3N4C5	102.86 (102.96)	C5N4C3N6	177.08 (176.79)
N4C5N1	110.18 (110.13)	H9N1C5N4	-179.83 (179.73)
C5N1N2	110.25 (110.33)	H9N1N2C3	179.70 (-179.80)
N4C3N6	121.82 (122.08)	N2N1C5N4	0.11 (0.21)

 

Table 2: Calculated structural parameters of 5(ATA): bond distances in Å and angles in°

B3LYP	5(ATA)
6- 311G++(d,p)
N1-N2	1.372 (1.372)	N1C5N6	124.10 (124.10)
N2-C3	1.315 (1.315)	C5N6H7	112.18 (112.18)
C3-N4	1.367 (1.367)	C5N6H8	116.22 (116.22)
N4-C5	1.321 (1.320)	H7N6H8	112.52 (112.52)
C5-N1	1.354 (1.354)	N1N2C3N4	0.31 (0.31)
C5-N6	1.380 (1.380)	N2C3N4C5	-0.44 (-0.44)
C1-H9	1.007 (1.007)	C3N4C5N1	0.37 (0.37)
N6-H7	1.011 (1.010)	N2N1C5N6	176.70 (176.70)
N6-H8	1.010 (1.010)	C3N4C5N6	-176.47 (176.47)
C3-H10	1.079 (1.079)	N4C5N6H7	-11.92 (-11.92)
N1N2C3	101.71 (101.71)	N4C5N6H8	-143.36 (-143.36)
N2C3 N4	115.67 (115.67)	N1N2C3H10	179.79 (179.79)
C3N4C5	102.86 (102.85)	H10C3N4C5	-179.92 (-179.92)
N4C5N1	109.82 (109.82)	H9N1C5N4	-175.18 (-175.18)
C5N1N2	109.94 (109.94)	H9N1N2C3	175.49 (175.49)
N4C5N6	126.00 (126.00)	N2N1C5N4	-0.21 (-0.21)
N2N1H9	120.00 (120.00)	H9N1C5N6	1.73 (1.73)
C5N1H9	129.89 (129.89)	C5N1N2C3	-0.06 (-0.06)
N2C3H10	121.57 (121.57)	N1C5N6H7	171.68 (171.67)
N4C3H10	122.77 (122.77)	N1C5N6H8	40.23 (40.23)

 

The values obtained are in good agreement with the previous datareported in the literature.^16,17 We can note that the molecular structures have a planar conformation with a partial electron delocalization between amino and triazolic groups.

Table 3: Energy and dipole moment

DFT/B3LYP 6-311G++(d,p)	3(ATA)		5(ATA)
	gas phase	aqueous phase	gas phase	aqueous phase
ET (u.a)	-297.70327	-297.71719	-297.70196	-297.71712
μ(Debye)	2.058	2.852	3.743	4.784

 

The analysis of Table 3 shows that 3(ATA) is energetically more stable than its isomer and solvated forms are slightly stabilized (respectively 0.38 and 0.41 eV). The values of the dipole moment μ vary in the same sense predicting a most important polarity for 5(ATA) and a strong electronic distribution.

Mulliken Charges

The calculation of charges on each atom allows us to determine the soil adsorption sites. The values obtained are reported in Table 4.

Table 4: Values of Mulliken atomic charges determined by DFT calculations

	3(ATA)		5(ATA)
Atom	Gas	Solution	Gas	Solution
N1	-0.213	-0.199	-0.183	-0.147
N2	-0.171	-0.242	-0.199	-0.285
C3	-0.049	-0.006	-0.067	-0.04
N4	-0.258	-0.34	-0.252	-0.334
C5	0.019	0.031	0.05	0.064
N6	-0.324	-0.38	-0.345	-0.385
H7	0.254	0.277	0.277	0.293
H8	0.252	0.278	0.236	0.279
H9	0.322	0.365	0.311	0.357
H10	0.17	0.216	0.171	0.197

 

It may be noted that the greatest values of the negative charges are carried by the atoms N1, N2, N4 and N6. Indeed, N2 and N4 have a pair of free electrons that would facilitate the adsorption on the ground surface. According to the results, the most appropriate site to create a binding is N4.The results obtained are in good agreement with those determined previously.²⁰

Molecular Electrostatic Potential Analysis

The molecular electrostatic potential is widely used as reactivity map providing very useful information for explaining structure-activity relationship, electronegativity, atomic net charges and chemical reactivity. Electronic isosurfaces obtained for the studied compounds, in gas phase and in solution, are reported in Figure 3.

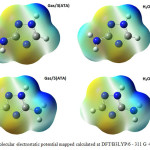Figure 3: Molecular electrostatic potential mapped calculated at DFT/B3LYP/6 – 311 G ++(d,p) level Click here to View figure

 

Regions where electrostatic potential is negative correspond to areas of high electronic density and, therefore, to a strong attraction between protons of the functional groups (carboxylic and phenolic) and humic substances. On these molecular surfaces, the involved regions are on N2 and N4 with respectively an isovalue of -0,053 and -0,071. The regions where values are positive (dark blue) have maximum repulsion and low electron density. More positive electrostatic potential regions are on triazole rings with a value of 0,106.

Frontier Orbitals Analysis

A characterization of electronic molecular orbital plays an important role in the chemical stability because they reflect the activity.^21,22 Electronic distributions frontiers molecular orbital (OMF’s) and their energetic difference ΔE are reported in Table 5.

Table 5: Energy of OMF’s in gaseous and solvated phases

DFT/6311G++(d,p)	3(ATA)		5(ATA)
	Gas	Solution	Gas	Solution
HOMO	-0.2301	-0.2382	-0.2432	-0.2461
LUMO	-0.0139	-0.0088	-0.0277	-0.0085
ΔE LUMO – HOMO	0.2162	0.2294	0.2155	0.2376

 

The frontiers molecular orbital, determined in gaseous and aqueous phases, are plotted in Figure 4.

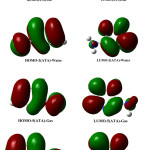Figure 4: Schematic representation of the frontier orbitals for 3(ATA) and 5(ATA) in gaseous and water phases Click here to View figure

 

It can be seen that the HOMO is delocalized over the amino group and on the double bonds of the triazole ring while LUMO is over C5-N4 bond and on each heteroatom. The analysis shows that solvation stabilizes slightly molecular orbital and leads to similar results. We can conclude that the compounds are less reactive in solvated phase.

Global and Local Reactivity Descriptors

The general behaviour of amitrole may be analysed using the global reactivity parameters derived from DFT. The results obtained previously served for determination of electronegativity (χ), electronic chemical potential (μ), hardness (η), softness (S), global electrophilicity index (ω) defined through the following equations where I and A represent respectively ionization potential and vertical electronic affinity.

χ = (I + A)/2                                         (1)

μ = − χ                                                 (2)

η= (I – A)                                             (3)

S = 1/2η                                               (4)

ω = μ²/2η                                             (5)

The results obtained (values in solution are in parentheses), allowing global reactivity affinities description, are grouped in Table 6.

Table 6: Global Reactivity Descriptor

Compound	χ (kJ/mol)	µ (kJ/mol)	η (kJ/mol)	S (kJ/mol)	ω (kJ/mol)
3(ATA)	320.311 (324.157)	-320.311 (-324.157)	567.791 (602.263)	0.00089 (0.00083)	90.3494 (87.2659)
5(ATA)	355.611 (334.161)	-355.611 (-334.161)	565.979 (623.793)	0.00088 (0.00080)	111.7171 (89.5035)

 

The values of hardness for both compounds are higher in solution leading to greater stability. With respect to ω electrophilic character index, the value obtained for the 5(ATA) isomer indicates a better reactivity.

In addition to the global reactivity parameters, it is essential to define local reactivity descriptors²³ as the Fukui Function which can be expressed as:

where ρ(r) is the electronic density.

However, due to the discontinuity of the electron density with respect to the number of electrons (N), this approximation leads to Fukui functions respectively named f_k⁺, f_k^– for electrophilic and nucleophilic attacks²⁴ evaluated through the following equations:

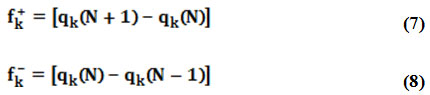
where q_k is the atomic charge evaluated from Mulliken population at the k^th atomic site in the neutral (N), cationic (N+1) or anionic (N-1) chemical species.

The use of local indices of Parr (ω_k and N_k) allows us to predict the most favorable chemical bond formation.They are given respectively by the expressions:

The results obtained for the different parameters are grouped in Tables 7 and 8.

Table 7: Local reactivity descriptors of 3(ATA).

3(ATA) – Gas	qk (N)	qk (N +1)	qk (N-1)	fk+	fk–	ωk	Nk
N1	-0.36475	-0.39667	-0.28576	0.03192	0.07899	0.02989	0.24590
N2	-0.38191	-0.3983	-0.15725	0.01639	0.22466	0.01535	0.69938
C3	0.50427	0.49351	0.53019	0.01076	0.02592	0.01007	0.08069
N4	-0.54675	-0.57762	-0.45809	0.03087	0.08866	0.02890	0.27600
C5	0.21572	0.19372	0.30435	0.022	0.08863	0.02060	0.27591
N6	-0.80286	-0.835	-0.49753	0.03214	0.30533	0.03009	0.95051
H7	0.38631	0.28991	0.43228	0.0964	0.04597	0.09026	0.14311
H8	0.38491	0.26716	0.4266	0.11775	0.04169	0.11025	0.12978
H9	0.4069	0.04049	0.45361	0.36641	0.04671	0.34307	0.14541
H10	0.19816	-0.07719	0.25162	0.27535	0.05346	0.25781	0.16642
3(ATA)-water	qk (N)	qk (N +1)	qk (N -1)	fk+	fk–	ωk	Nk
N1	-0.3528	-0.41372	-0.27291	0.06092	0.07989	0.05508	0.23118
N2	-0.41225	-0.43499	-0.17626	0.02274	0.23599	0.02056	0.68289
C3	0.5063	0.46662	0.53986	0.03968	0.03356	0.03588	0.09711
N4	-0.59079	-0.60479	-0.49886	0.014	0.09193	0.01266	0.26602
C5	0.22912	0.21083	0.31496	0.01829	0.08584	0.01654	0.24840
N6	-0.81809	-0.87377	-0.4954	0.05568	0.32269	0.05034	0.93377
H7	0.39557	0.25579	0.43647	0.13978	0.0409	0.12638	0.11835
H8	0.39558	0.15256	0.4338	0.24302	0.03822	0.21972	0.11060
H9	0.43012	0.07021	0.46489	0.35991	0.03477	0.32541	0.10061
H10	0.21724	0.17127	0.25346	0.04597	0.03622	0.04156	0.10481

 

Table 8: Local reactivity descriptors of 5(ATA).

5(ATA) – Gas	qk (N)	qk (N +1)	qk (N -1)	fk+	fk–	ωk	Nk
N1	-0.40231	-0.43416	-0.35524	0.03185	0.04707	0.03688	0.12971
N2	-0.32539	-0.3548	-0.07446	0.02941	0.25093	0.03405	0.69150
C3	0.18362	0.17032	0.26671	0.0133	0.08309	0.015400	0.22898
N4	-0.56611	-0.59146	-0.4235	0.02535	0.14261	0.02935	0.39300
C5	0.54416	0.54136	0.59443	0.0028	0.05027	0.00324	0.13853
N6	-0.80339	-0.86809	-0.56337	0.0647	0.24002	0.07491	0.66144
H7	0.39632	0.26088	0.4358	0.13544	0.03948	0.15682	0.10880
H8	0.37822	-0.09937	0.42393	0.47759	0.04571	0.55299	0.12597
H9	0.40299	0.20531	0.44873	0.19768	0.04574	0.22889	0.12605
H10	0.19189	0.17002	0.24697	0.02187	0.05508	0.02532	0.15179
5(ATA) – water	qk (N)	qk (N +1)	qk (N -1)	fk+	fk–	ωk	Nk
N1	-0.39481	-0.42127	-0.32348	0.02646	0.07133	0.02454	0.19106
N2	-0.35957	-0.37533	-0.12699	0.01576	0.23258	0.01462	0.62296
C3	0.178	0.17768	0.26252	0.00032	0.08452	0.00030	0.22639
N4	-0.60515	-0.61266	-0.46112	0.00751	0.14403	0.00697	0.38578
C5	0.55794	0.55296	0.59654	0.00498	0.0386	0.00462	0.10339
N6	-0.80787	-0.93647	-0.52706	0.1286	0.28081	0.11929	0.75214
H7	0.4028	0.33657	0.43716	0.06623	0.03436	0.06144	0.09203
H8	0.40269	-0.264	0.4412	0.66669	0.03851	0.61845	0.10315
H9	0.42781	0.34746	0.46316	0.08035	0.03535	0.07454	0.09468
H10	0.19813	0.19507	0.23806	0.00306	0.03993	0.00284	0.10695

 

It may be noted that the distributions of the most reactive sites of this herbicide are located on N2 and N4 confirming the values obtained with the Fukui indices (Figure 5).

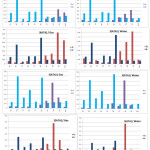Figure 5: Values of the Fukui function for 3(ATA) and 5(ATA) in gaseous and water phases  Click here to View figure

 

From all the results detailed above, it can be concluded that an electrophilic attack will preferably occur at the level of the triazole ring, more particularly on the N2 atom for the 5(ATA) in the two phases. Protonation of 3(ATA) will take place certainly at the level of N4 since this herbicide is stable in solution. These results suggest that 5(ATA) is always the most reactive and 3(ATA) is the most stable in solution.

Conclusion

In the present work, the geometries of studied compounds were optimized with DFT/B3LYP methods using 6-311G++(d,p) basis set in gas and in aqueous phase. The molecular structural parameters were obtained and the Mulliken charges calculated were presented. Information on charge density distribution and structure-activity relationship were obtained by mapping electron density isosurfaces with electrostatic potential. Molecular Orbital’s study reveals the intramolecular charge transfer through the conjugated system. Determination of chemical reactivity of 3(ATA) and 5(ATA) in both phases employing global and local reactivity parameters indicates that 5(ATA) is the most reactive while 3(ATA) is the most stable in ground soil. The Fukui function values suggest that electrophilic attacks on herbicides would be on N4 in aqueous conditions. The present quantum chemical study indicated that hydrogen bonds could have occurred between the soil and the organic molecules and determined the available active groups.

Acknowledgements

The authors thank AMCT for Gaussian program.

References

1. Sellamuthu, R. 2014. Amitrole, Author Vitae Encyclopedia of Toxicology (Third edition), 203-205.
   CrossRef
2. Pozdnyakov, I.P.; Sherin, P.S.; Salomatova, V.A.; Parkhats, M.V.; Grivin, V.P.; Dzhagarov     B.M.; Bazhin N.M.; Plyusnin V.F. Environ Sci.Pollut.Res.Int.2017.  doi:10.1007/s11356-017-8580-x.
   CrossRef
3. Chicharro, M.; Bermejo, E.; Moreno, M.; Sanchez, A.; Zapardiel, A.; Rivas, G. Electroanalysis.  2005, 17, 476-482.
   CrossRef
4. Baglieri,A.;Vindrola,D. http://www.chembioagro.com/content/1/1/9/-ins2; Gennari,M. http://www.chembioagro.com/content/1/1/9/- ins1; Negre, M. Chemical and Biological Technologies in Agriculture. 2014. 1-9.
5. Tazi, R.; El Hasiní, S.; EL YacoubiKhouja, H.; Zaydoun, S.; Zrineh, A.; El Azzouzi, M. Research Journal of Pharmaceutical, Biological and Chemical Sciences RJPBCS. 2015, 6, 227-234.
6. El Fadil, I.; Tazi, R.; Ouezzani, I.; Altarawneh, R.; Batarsehand, M.;  El Azzouzi, M. Research Journal of Pharmaceutical, Biological and Chemical Sciences RJPBCS. 2015, 6, 1463-1472.
7. McCarthy, J.F. ; Jimenez, B.D. Environ. Sci. Technol. 1985, 19, 1072-1076.
   CrossRef
8. Gauthier, T. D.; Shane, E. C. ; Guerin, W. F. ; Seitz, W. R. ; Grant C. L. Environ. Sci. Technol. 1986, 20, 1162-1166.
   CrossRef
9. Mill, Th. Chemosphere. 1999, 38, 1379-1390.
   CrossRef
10. (a) Bradley, J.D.; Gerrans, G.C. J. Chem. Educ. 1973, 50, 463 (b) Olbrich, C.; Strümpfer, J.; Schulten, K.; Kleinekathöfer, U. J. Phys. Chem. Lett. 2011, 2, 1771-1776 (c) Fukui,K. ; Yonezawa,T. ;  Shingu,H. J. Chem. Phys.1952, 20, 722-725.
    CrossRef
11. (a) Becke,A.D. J. Chem. Phys. 1993, 98, 5648–5652. (b) Becke,A.D.  Phys. ReV A. 1988, 38, 3098-3100  (c) L e e, C.;  Y a n g, W. ; P a r r , R .G. ; Phys. ReV. B. 1988, 3, 785-789.
    CrossRef
12. Frisch, M.J.;  Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.;  Robb, M.A.; Cheeseman, J.R.; Montgomery Jr, J.A.; Vreven, T. ; Kudin, K.N.;  Burant, J.C.;  Millam, J.M.; Iyengar, S.S.;  Tomasi, J.; Barone, V.; Mennucci, B.; Cossi, M.; Scalmani, G.; Rega, N.; Petersson, G.A.;  Nakatsuji, H.; Hada, M.; Ehara, M.; Toyota, K.; Fukuda, R.; Hasegawa, J.; Ishida, M.; Nakajima, T.; Honda, Y.; Kitao, O.; Nakai, H.; Klene, M.; Li, X. ; Knox, J.E.; Hratchian, H.P.; Cross, J.B.; Bakken, V.; Adamo, C.; Jaramillo, J; Gomperts, R.; Stratmann, R.E.; Yazyev, O.; Austin, A.J. ; Cammi, R.; Pomelli, C.;  Ochterski, J.W.;  Ayala, P.Y.; Morokuma, K.; Voth, G.A.; Salvador, P.; Dannenberg, J.J.; Zakrzewski, V.G.; Dapprich, S.; Daniels, A.D.; Strain, M.C.; Farkas, O.; Malick, D.K.; Rabuck, A.D.; Raghavachari, K.; Foresman, J.B.; Ortiz, J.V. ; Cui, Q.; Baboul, A.G.; Clifford, S.; Cioslowski, J.; Stefanov, B.B.; Liu, G.; Liashenko, A.; Piskorz, P.; Komaromi, I.; Martin, R.L.; Fox, D.J. ; Keith, T.; Al-Laham, M.A.; Peng, C.Y.; Nanayakkara, A.; Challacombe, M.; Gill, P.M.W. ; Johnson, B.; Chen, W.; Wong, M.W.; Gonzalez, C.; Pople, J.A.; Gaussian 03, Revision D, Gaussian,Inc., Wallingford, CT, 2004.
13. Dennington II, R.; Keith, T.; Millam, J.; Eppinnett, K.; Hovell, W.L.; Gilliland, R.; GaussView, Version 3.0.9, Semichem Inc., Shawnee Mission, KS, 2003.
14. Becke,A.D. Journal of Chemical Physics.1993, 98, 5648‑5652.
    CrossRef
15. Keresztury,G.; Holly,S.; Besenyei,G.; Varga,J. ; Wang,A.; Durig, J. R. SpectrochimicaActa Part A: Molecular Spectroscopy. 1993, 49, 2007‑2026.
    CrossRef
16. Reed,A. E.; Curtiss,L. A.; Weinhold,F.Chem. Rev. 1988, 88, 899-926.
    CrossRef
17. Reed,A. E.; Weinstock,R. B.; Weinhold,F. Journal of Chemical Physics. 1985,83, 735‑746.
    CrossRef
18. Barone, V.; Cossi, M.; Tomasi, J. J. Chem. Phys. 1997, 107, 3210-3221.
    CrossRef
19. (a) GilchristT.I.; Gymer.G.E. Advances in Heterocyclic Chemistry.1974, 16, 33-85 (b) Pagacz-Kostrzewa, M.; Bronisz, R.; Wierzejewska, M. Chemical Physics Letters. 2009, 473, 238-246 (c) Shojaie,F.Jordan Journal of Chemistry. 2015, 10, 161-178 (d) Yankova,R.; Radev,L. Journal of Materials and Chemistry, 2015, 5, 25-30 (e) Yankova,R.; Avramov,S. Journal of Multidisciplinary Engineering Science Studies. 2016, 2, 569-573.
    CrossRef
20. Dżygiel,A.; Masiukiewicz,E.; Rzeszotarska,B. J. Agric. Food Chem. 2002, 50, 1383-1388.
21. Marakchi,K.; Ghailane,R.; Kabbaj,O.K.; Komiha,N. J. Chem. Sci.2014, 126, 283-292.
    CrossRef
22. Salah,M.;  Komiha,N.;  Kabbaj,O.K;  Ghailane,R.;  Marakchi,K.; Journal of Molecular Graphics and Modelling. 2017, 73, 143-151.
    CrossRef
23. Parr,R.G. J. Am. Chem. Soc. 1984, 106, 4049-4050.
    CrossRef
24. Borgoo,A.; Tozer,D.J.; Geerlings,P. J. Chem. Phys. 2009, 11, 2862-2868.
    CrossRef

---

This work is licensed under a Creative Commons Attribution 4.0 International License.