Spectrophotometric Determination of Kinetic Constants of Fe (Iii)-Amines’s Charge Transfere Complexes

Introduction

Many electronic spectrophotometric studies have been reported in the coordination chemistry field on complexes. ^[1-6] Generally, these complexes were obtained by using different electron donors with various organic, ^[7-10] or metallic electron acceptors ^[11-13] in polar and non-polar solvents. In this work, the formation of complexes were discussed and different parameters concerning their behaviour in methanol solution at 25°C were calculated. Usually the formation constants (K^AD) and molar extinction coefficients (ε^AD) of complex were calculated using the equations of Benesi-Hildebrand, Scott and Foster-Hammick-Wardley ^[14-16] (equations 1, 2 and 3) respectively by using the obtained experimental data:

[A₀] /A = 1/ K^AD ε^AD [D₀] + 1 / ε^AD                       (1)

[D₀] [A₀] /A = [D₀] / ε^AD + 1 / ε^AD K^AD               (2)

A / [D₀] = – K^AD A + K^AD ε^AD [A₀]                  (3)

Where A is, the absorbance of the complex band, [A_o] and [D_o] are the initial concentrations of the electron acceptor and the electron donor respectively. K^AD is the formation constant of the complex in solution, while ε^AD represents the molar extinction coefficient of complex. The charge transfer complexes may also be studied by evaluating their electronic absorption band energies. The ionization potentials of electron donors can be obtained from charge transfer absorption bands by using different approaches as in the following equations ^{[8, 17-19]}:

This paper is dedicated to the memory of the late Professor Ali Hassoun Al-Taiar

h. uAD = ID – C1 + C2/ ID - C1   (4)

h. u_AD   =   α I^D+ b                                    (5)

I^D = 4.39 + 0.857 hu_AD                          (6)

I^D   = 5.1 + 0.7 hu_AD                                 (7)

Where hu_AD is the absorption band energy of charge transfer complex, and I^D is the ionization potential of the electron donor. C₁= 5.2 eV, C₂ = 1.5 eV, a = 0.67 or 0.87, b = −1.9 or −3.6.

Several kinetics parameters of association of electron donors with electron acceptors been reported. ^{[20, 21]}

Experimental

n-Butyl amine, cyclohexyl amine, phenylhydrazine and Ferric chloride with a purity of 99.8% obtained from Merck have been used without further purification. Methanol (Fluka) was distilled before using. The UV-visible spectra were measured using a Pye Unicam SP8-100 spectrophotometer. The complexes were prepared by mixing variable amounts of donor solutions with an acceptor solution in methanol. The donor concentrations used ranged between 1.10⁻³ and 4.10⁻⁹ mol/L while the acceptor concentration remained constant (1.10⁻³ mol/L).

Results and Discussion

In this work, we investigated the kinetics of the equilibrium between n-butyl amine, cyclohexyl amine, phenylhydrazine and Ferric chloride and their complexes in methanol at 25°C. Also, calculating the equilibrium constants and the orders of the reaction as well as the rate constants of the forward and the reverse reactions. Moreover, the half-life period of the reactions also obtained. In addition, the ionization potentials of the electron donors calculated spectroscopically. The reason for choosing the amines as complexing agents is that their reaction with Fe (III) ion is slow and easy to measure. The importance of all calculations in this work is to prove the validity of Scott and Foster-Hammick-Wardley equations for these reactions. Another major objective of this study was the calculation of the formation constants of the complexes. In principle, the analysis of the UV/Visible spectrometric method using the Benesi-Hildebrand’s equation, later modified by Scott, permits these determinations for complexes of 1:1 type, for which the proposed equation is linear. In addition, this method provides an easier and direct determination of iron without any extraction or heating. Finally, for additional works, some results could be obtained to give complementary  parameters, such as pH of  the solution, nature of solvent and temperature in the complexation reactions of Fe (III), and hence to accomplish determination of iron in different water  samples. Table 1 shows the symbols and structures of donors (D_i) and acceptor (A) FeCl₃.

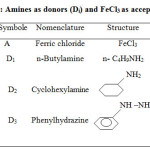Table 1: Amines as donors (Di) and FeCl3 as acceptor (A)    Click here to View table

 

The UV maximum wavelength absorption band of donors, acceptor and complexes are shown in Table 2. All maximum absorption bands of the acceptor and donors disappeared and the new absorption bands of the complexes are located in the visible region probably due to the complete transfer of electrons from the donor, which has ionic properties, to the unfilled orbital of the transition ferric ion.

Table 2:  Maximum wavelength of the absorption bands of the donor, acceptor and complex (AD) in methanol at 25°C

Compound Donors	A lmax (n.m)	D lmax (n.m)	A D lmax (n.m)
D1	275	277	340
D2	275	277	406
D3	275	273	361

 

E_AD = 1243.667 / l_max   (e.V)                           (8)

DG⁰= – RT ln K^AD   =   – 2.303RT log K^AD       (9)

Where, DG⁰is the free energy of complex (in kJmol^-1), R the gas constant (8,314 J mol^-1 K^-1), T the temperature in Kelvin.  The value of ionization potentials I_D of the donors calculated using equations 4 – 6  are equals to,  7.131,  7.848, 7.100 eV for D₁, D₂ and D₃ respectively.

For phenylhydrazine-Fe complex it is observed that equation (2) give the better straight line (R² = 0.999) when [D₀].[A₀]/A was plotted against [D₀] with support the formation of charge transfer complex (Figure 1).

Figure 1:  Scott plots of charge transfer complex of phenylhydrazine with Fe(III) in methanol at 25 °C.   Click here to View figure

 

Thus, ε^AD and K^AD are easily determined. The regression equation describing the line obtained from Scott plot is given by:

[D₀].[A₀]/A  = 0,021.10^-6 + 4,25 10⁺²[D₀]

In this plot the slop and intercept equals 1/ ε^AD and 1/ ε^AD K^AD respectively. The formation constant and molar coefficient of the AD complex were calculated and shower in Table 3. The others coefficients are calculated from equation (1).

Table 3 gives the values of ε^AD and K^AD, absorption band energy (E) and free energy (DG) of the complex reaction in methanol at different donor concentrations at 25°C. When equation (2) is used and plotted, the value K^AD and ε^AD for the complex phenylhydrazine-Fe (AD₃) complex obtained as shown in figure 1 and table 3.

These values of ε^AD are 2488 L.cm⁻¹.mole⁻¹ while those of K^AD are 4246 L.mole⁻¹.

From the values of ε^AD show that lower amounts of donor are more sensitive to the absorption than higher concentrations. These high values of K^AD may show the high stability of the complex in methanol solutions.

Table 3: Values of formation constant (K^AD), molar extinction coefficient (ε^AD), energy of the absorption band (E) and free energy change (DG) of the complex reaction in methanol at 25°C:

	Complex AD1	Complex AD2	Complex AD3
KAD (l/mol)	709.06	473	4246
εAD (l/mol.cm)	2150.70	693.02	2488
E (ev)	3.6483	3.0552	3.435
DE (ev)	5.36 x 10-3	3.762 x 10-3	4.765 x 10-3
-DG  (kj/mol)	16.37	15.56	20.53

 

The reaction of phenylhydrazine as an example with ferric ion is estimated to be a second order reaction as in Figure 2 and Table 4.

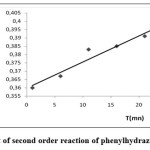Figure 2:  Plot of second order reaction of phenylhydrazine-Fe complex   Click here to View figure

 

The rate constant (k₁) of the forward reaction was calculated from the graph and the value obtained was 0,97 x 10^-3 L.mol^-1.min^-1 from which the half-life (t_1/2) of 206 min. was obtained. Using the values of the equilibrium constant shown in Table 3, the rate constant of the reverse reaction (k₋₁) was calculated to be 0,23 x 10^-6 L.mol^-1.min^-1. Since it is none that K^AD = k₁/k_-1 (see table 3)

Table 4:  Results of the kinetic studies

Symbol	Complex	Order of reaction	Rate* constant k1.103	Rate* constant k-1.106	t1/2 (min)
AD1	n-Buthylamine-Fe	0	2.70	3.81	5330
AD2	Cyclohexamine-Fe	1	4.03	8.52	1720
AD3	Phenylhydrazine-Fe	2	0,97	0.23	206

*the unit of the rate constant k depends on the order of the reaction.

Order 0: (k₁) = mol. L^-1.min.^-1 , Order 1: (K₁) = min.^-1, Order 2: (K₁) = L. mol.^-1.min.^-1

These values of K^AD, k₁ and k₋₁ show that the stability of phenylhydrazine-Fe complex is very high and the forward reaction rate is much higher than the reverse reaction rate. K^AD values of the complexes vary between 473 – 4246 l/mol. These results are in accord that iron ion/ Phenylhydrazine complex is more stable.

Conclusions

Charge transfer complex (AD) was obtained and the colored reaction of phenylhydrazine with iron (III) was studied using Scott equation and the spectrometric UV-visible data. The obtained results indicate the formation of 1:1 charge transfer complex. Phenylhydrazine is one of the sensitive spectrophotometric reagents for iron. The molar absorptivity of the complex reaches 2488 L.cm^-1.mol^-1. The determination of some complexation parameters shows that the reaction is instantaneous. The association constant Fe (III)-phenylhydrazine and Gibbs free energy are 4246 L.mol^-1 and -20.53 kJ.mol^–1, respectively.

Acknowledgments

Thanks are due to Professor Anis Al-Najjar of Basrah University, Iraq, for scientific comments and to Mrs. Karima Abed for arranging references.

References 

1. Gopala Krishna D.; Devi C.K.  World J.Pharmacy and Pharmaceutical Sci. 2014, 3(2), 1260-1266.
2. Cano-Guzmán C.F.; et al.  J Textile Sci Eng. 2014, 4:155. doi: 10.4172/2165-8064.1000155
   CrossRef
3. Adikwi M.U.; Ofokansi K.C.; Attama A.A.  Chem. Pharm. Bull. 1999, 47(4): 463-466.
   CrossRef
4. Refat M.S.; Sadeek S.A.  Can. J. Of anal. Sc. and spectr. 2006, 51(6): 312-322.
5.  fazuddin T.;  Biswanath M.  Proc. Indian Acad. Sci. (Chem. Sci.). 2000, 112(4): 475–485.
6. Khan  I.M; Ahmad  A.  Spectrochim. Acta, Part A. 2009, 73: 966- 971.
   CrossRef
7. Dehghani  H.; Sahba  R.; Afrooz  M.; Mollaei  H.  Journal of the Chinese Chemical Society. 2010, 57: 690-695.
   CrossRef
8. Gao F.; Yang Y.Y.  Chines Chemical Letters. 1999, 10(22): 1041-1044.
9. Saeed A. A. H.; Othman S.A.W.;  Al-Jhalil  K. A.; Atto  A. T.  Can. J. Spectrosc. 1981, 2638-2645.
10. Ishaad M.K.; Ahmad A.; Oves M.  Spectrochim. Acta Part A. 2010, 77: 1059-1064.
    CrossRef
11. Sajid H.; Guzar J.; Qin Han I.   J. appl. Sc. 2008, 8(13): 2480- 2485.
12. Al-Taiar A. H.; Shubber S.H.; Megharbi A.  Turk. J. Chem. 2002, 26: 351- 355.
13. Shahdousti P.; Aghamohammadi M.; Alizahed N.  Spectrochim. Acta Part A, 2008, 69, 1195-1200.
    CrossRef
14. Benesi H. A.; Hilderbrand J. H.  Amer  J.  Chem. Soc. 1949, 71: 2703.
    CrossRef
15. Scott R. L.  Proc. Int. Conf. On Co-ordination compounds, Amsterdam 1955.
16. Foster R.; Hammick D. L.; Wardley A.A.  J. Chem. Soc. 1953, 3817-3820.
    CrossRef
17. Chan R.K.; Liao S.C.  Can. J.  Chem. 1970, 48: 299-305.
    CrossRef
18. Singh N.; Ahmad A. Can. J. of Anal. Sci.  Spectros. 2009, 54(1):  11-22.
19. Subhani M.S.; Aslam S.; Qureshi R.; Rahman A.  J. Chem. Soc. Pak. 2008, 30(2):232-236.
20. Absil A. C.; Buxeraud J.;  Raby C. Can. J. Chem. 1984, 62: 1807- 1811.
    CrossRef
21. Subhani M.S.; Bhatti N. K.; Mohammad M.; Khan A. Y.  Turk J.  Chem. 2000, 24(2): 223- 230.

---

This work is licensed under a Creative Commons Attribution 4.0 International License.