QM/MM & Monte Carlo simulation of single wall nano tube carbon SWNT (15, 15) binding with thymine dimer

Introduction

The carbon nanotube (CNT) is a representative nano-material. CNT is a cylindrically shaped carbon material with a nanometric-level diameter [1-8].

Its structure, which is in the form of a hexagonal mesh, resembles a graphite sheet and it carries a carbon atom located on the vertex of each mesh. The sheet has rolled and its two edges have connected seamlessly [9-15].

Although it is a commonplace material using in pencil leads, its unique structure causes it to present characteristics that had not found with any other materials. CNT can be classified into single-wall CNT, double-wall CNT and multi-wall CNT according to the number of layers of the rolled graphite [16, 17].

The type attracting most attention is the single-wall CNT, which has a diameter deserving the name of “nanotube” of 0.4 to 2 nanometers. The length is usually in the order of microns, but single-wall CNT with a length in the order of centimeters has recently released [18,19].

CNT can be classified into single-wall CNT, double-wall CNT and multi-wall CNT according to the number of layers of the rolled graphite. The type attracting most attention is the single-wall CNT, which has a diameter deserving the name of “nanotube” of 0.4 to 2 nanometers [20].

The length is usually in the order of microns, but single-wall CNT with a length about centimeters have recently released. The extremities of the CNT have usually closed with lids of the graphite sheet [21-23].

The lids consist of hexagonal crystalline structures (six-membered ring structures) and a total of six pentagonal structures (five-membered ring structures) placed here and there in the hexagonal structure [24]. The first report by Iijima was on the multi wall form, coaxial carbon cylinders with a few tens of nanometers in outer diameter [25]. Two years later single walled nanotubes were reported [26,27]. SWCNTs have considered as the leading candidate for nano device applications because of their one-dimensional electronic bond structure, molecular size, and biocompatibility, controllable property of conducting electrical current and reversible response to biological reagents hence SWCNTs make possible bonding to polymers and biological systems such as DNA and carbohydrates[28-35].

So, The structure of SWCNT as well as their dipole moments and relative energies have been studied by molecular dynamics simulation and quantum mechanics calculations within the Onsager self – consistent reaction field (SCRF) model using a Hartree-Fockmethod (HF) at the BLYP/6-21G*level and the structural stability of considered nanotube in different solvent media and temperature have been compared and analyzed.We investigate effects of water, methanol and a mixture of them on interaction of Thymine-dimer with SWCNT, utilizing these force fields too [36-40].

The interaction of Thymine-Dimer with open-end of SWCNT in gas phase, water and methanol have been processed applying the abinitio calculations.

The calculations have been done with the GAUSSIAN 98 program according to DFT theory at the HF/6-31g* level. Gibbs free energy,enthalpy,entropy and dipole moment values are compared in gas phase, water and methanol, in this research[41]. Thus, by utilizing a Hartree-Fock method, we studied the effects of different  solvents on interaction of Thymine-dimer with  open-end of  SWCNT within the onsager  self-consistent reaction field (SCRF) model,  and the temperature effects on the stability of the interaction between Thymine-dimer and single-walled carbon in various solvents[42-44].

Theoretical Background

A Normal mode

A harmonic oscillator approximation is most widely used for computing molecular vibrational frequencies because more accurate methods require very large amounts of CPU time. Frequencies computed with the Hartree-Fock approximation and a quantum harmonic oscillator approximation tends to be 10% too high due to the harmonic oscillator approximation and lack of electron correlation [45, 46 and 47]

Many studies have shown that the carbon nanotubes possess remarkable mechanical and physical properties leading to many potential applications such as fluid transport, fluid storage at nanoscale, and nano-devices for drug delivery Since controlled experiments at the nanometer scale are very difficult, the simulation techniques have been widely and successfully used to investigate the mechanical property, wave propagation and resonant frequency [48].

The vibration of molecules is best described using a quantum mechanical approach. A harmonic oscillator does not exactly describe molecular vibrations. Bond stretching is better described by a Morse potential and conformational changes have sine-wave-type behavior. However, the harmonic oscillator description is very useful as an approximate treatment for low vibrational quantum numbers [49-55].

B:DFT Concept and Monte Carlo simulation

Because of imperfections of the force field, this lowest energy basin usually does not correspond to the native state in most cases, so the rank of native structure in those decoys produced by the force field itself is poor.

In density function theory the exact exchange for a single determination is replaced by a more general expression the exchange correlation functional, which can include terms accounting for both exchange energy and the electron correlation, which is omitted from Hartree-Fock theory:

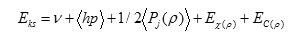
Where E_k(p) is the exchange function and E_c(p) is the correlation functional.

The Metropolis implementation of the Monte Carlo algorithm has been developed by studying the equilibrium thermodynamics of many-body systems. Choosing small trial moves, the trajectories obtained applying this algorithm agree with those obtained by Langevin’s dynamics [59]. This is understandable because the Monte Carlo simulations always detect the so-called “important phase space” regions which are of low energy [60].

The correlation function of Lee, Yang and Parr is includes both local and non-local term [61-64].

C: Simulation of Langevin dynamics

The Langevin equation is a stochastic differential equation in which two force terms have been added to Newton’s second law to approximate the effects of neglected degrees of freedom [65]. These simulations can be much faster than molecular dynamics.The molecular dynamics method is useful for calculating the time dependent properties of an isolated molecule. However, more often, one is interested in the properties of a molecule that is interacting with other molecules [66].

Calculation details

In general, the electron distribution around a nucleus in a molecule is more spherically symmetric. Therefore, the size of electron current around the field, and hence the size of the shielding, will depend on the orientation of the molecule within the applied field B₀.

For chemical shielding (CS) tensor which describes how the size of shielding varies with molecular orientation?

The term “Ab Initio” is given to computations which are derived directly from theoretical principles, with no inclusion of experimental data. The most common type of ab initio calculation is called a Hartree Fock calculation (abbreviated HF), in which the primary approximation is called the central field approximation. A method, which avoids making the HF mistakes in the first place, is called Quantum Monte Carlo (QMC). There are several flavors of QMC variational, diffusion and Green’s functions. These methods work with an explicitly correlated wave function and evaluate integrals numerically using a Monte Carlo integration. These calculations can be very time consuming, but they are probably the most accurate methods known today. In general, ab initio calculations give very good qualitative results and can give increasingly accurate quantitative results as the molecules in question become smaller [67].

There are three steps in carrying out any quantum mechanical calculation in HyperChem 7.0 program package [68].First, prepare a molecule with an appropriate starting geometry. Second, choose a calculation method and its associated options. Third, choose the type of calculation with the relevant options.

DFT is based on a theorem due to Hohenberg and Kohn, which states that all ground state properties are functions of the total electronic charge density ρ(r).There are several different DFT functional available differing primarily in the choice of the basis set functions, in which, the electronic wave functions are expanded and the scheme of integration.[69,70].

The Becke’s three parameter exact exchange functional (B3) combined with gradient corrected correlation functional of Lee–Yang–Parr (LYP) have been employed to calculate energy, dipole moment, charge distribution and thermo chemical data and  NMR  parameters by implementing the 6-31G,STO-3G basis sets.  All the NMR shielding parameters were calculated supposing gauge-included atomic orbital (GIAO) method.

NMR spectroscopy is a research technique that exploits the magnetic properties of certain atomic nuclei to determine physical and chemical properties of atoms or the molecules in which they are contained. It relies on the phenomenon of nuclear magnetic resonance and can provide detailed information about the structure, dynamics, reaction state, and chemical environment of molecules. Ab initio calculation of nuclear magnetic shielding has become an indispensable aid in the analysis of molecular structure and accurate assignment of NMR spectra of compounds.

NMR is based on the quantum mechanical property of nuclei. The chemical shielding refers to the phenomenon, which is associated with the secondary magnetic field created by the induced motions of the electrons that surrounding the nuclei when in the presence of an applied magnetic field.

Calculations of nucleus-dependent and -independent chemical shifts were carried out using the gauge-invariant atomic orbital (GIAO) approach.

Results and discussion

We used different force field to determination of energy and other type of geometrical parameters, on the particular SWCNT, Because of the differences among force fields, the energy of a molecule calculated using two different force fields will not be the same (Fig.1).

In the process of investigating the combination of Thymine dimer coupling and CNT to achieve more information about these important gene systems, we attempted to construct different sequences of DNA (Thymine dimmer section) include three nucleotides with these sequences: TTG, TGT, TTT and link them individually to outer surface of SWCNT to develop practical application of Thymine dimmer coupling inside the SWNTs. These coupling have been performed between N atom of NH2 groups of nucleotide T in TTT sequence of DNA, nucleotide T in TGT and TTG sequence of mRNA and nucleotide C in CAG sequence of mRNA with C atom in SWCNT. In Fig.2 and Fig 3 and Fig 4 the couplings between these sequences of DNA atoms with outer surface of SWNT have been displayed. Optimizations of these structures have been performed by using UFF and AM1and DFT [71].

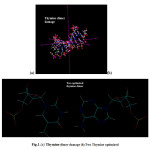Figure1:(a) Thymine dimer damage (b) Two Thymine optimized  Click here to View figure

 

So, it is not reasonable to compare the energy of one molecule calculated with a particular force field with the energy of another molecule calculated using a different force field. In this study difference in force field illustrated by comparing the energy of calculated by using force fields, MM+, Amber and OPLS. Theoretical energy values using different force fields, which is the combination of attraction van der Waals forces due to dipole-dipole interactions and empirical repulsive forces due to Pauli repulsion has been demonstrated in Table1 and Fig.2.Since the solute dipole moment induces a dipole moment in opposite direction in the surrounding medium.

Figure2: Thymine dimersurrounding with 500 H2O   Click here to View figure

 

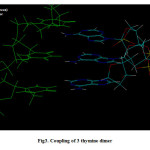Figure3: Coupling of 3 thymine dimer    Click here to View figure

 

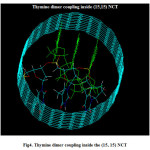Figure4: Thymine dimer coupling inside the (15, 15) NCT   Click here to View figure

 

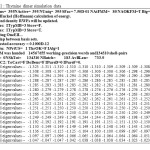Table1: Thymine dimer simulation data  Click here to View table

 

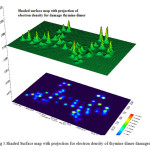Figure5: Shaded Surface map with projection for electron density of thymine dimer damaged   Click here to View figure

 

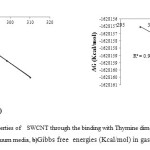Figure5:a)Thermodynamic Properties of   SWCNT through the binding with Thymine dimer at different temperatures using STO-3G basis set in vacuum media, b)Gibbs free  energies (Kcal/mol) in gas phase.  Click here to View figure

 

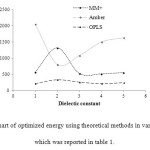Figure6: The chart of optimized energy using theoretical methods in various media, which was reported in table 1.  Click here to View figure

 

Also, the energies have compared to the gas phase total energy CNT at the B1LYP/3-21G level of theory and different solvents and the graph of energy values versus dielectric constant of different solvents has been displayed at considered temperatures in Fig.3.

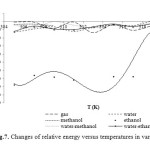Figure7: Changes of relative energy versus temperatures in various media.   Click here to View figure

 

Also, polarization of the medium in turn polarizes the charge distribution in the solvent. The Thymine dimer simulation datahas been reported in Table1.

One much more practical approach consists of calculating the molecular volume as defined through the contour of constant electron density(Fig 5), equating this (non-spherical) molecular volume to the radius of an (ideally spherical) cavity, and adding a constant increment for the closest possible approach of solvent molecules . This final approach used in Gaussian when the volume keyword was being used. Since, the influence between a molecule in solution and its medium can describe most simply by using Onsager model. In this model we have assumed that the solute is placed in a spherical cavity inside the solvent. The latter is described as a homogeneous, polarizable medium of dielectric constant. We performed our studies at B1LYP/3-21G gas phase geometry and water, methanol and ethanol surrounding single-walled carbon nanotube.

(SWCNT) and mixed of them either. The results obtained from Onsager model calculations illustrated using the energy difference between these conformers which quite sensitive to the polarity of the surrounding solvent. The solvent effect has been calculated using SCRF model.

Also, we measured the interaction between these codons and CNT by calculating two characteristics of demonstrated systems: total energy and dipole moment. The results of the optimized structures which were performed at the HF, B3LYP levels are reported in table1.The results represent that the energy between a specific codon coupled with carbon atoms of CNT was less than the other codons: CNTs complexes (Fig1-4).

we have inferred the Correlation between transportation of SWCNT through binding with thymine-dimer and the Gibbs free energy, entropy, enthalpy. According to the results, we can receive this formula:

ΔG = ΔH – T ΔS                         ΔG = 0.07 – 0.13 T

R² = 0.99

Accordingly, it can be seen whilst the temperature increases the Gibbs free energy decreases so we have a constant system. On the other hand with this kind of interaction, we could catch a good result for R²  because it’s near to number 1.

The GIAO (gauge independent atomic orbital) approximations of NMR calculations for Structural study of the combined systems of mRNA and CNT to obtain nuclei Magnetic Resonance Parameters via Density Functional Theory (B3LYP) and Hartree Fock methods with STO-3G and 3-21G basis sets have been performed in gas phase too.

The results of NMR calculations consist of chemical shift anisotropy asymmetry (η), isotropy ( σiso) , anisotropy (σaniso), and Δσ and chemical shift tensor (δ)parameters have been obtained and reported in Tables 3.

References

1. Massimo Fusaro.Quantum Matter, 2014, 3, 481-487.
2. Micheal Arockiaraj. Rev. Theor. Sci, 2014 2, 261-273.
3. Martin Bohlén.; Kim Bolton.Quantum Matter, 2014 3, 339-343.
4. Monajjemi, M.;Najafpour,J. Fullerenes, Nanotubes, and Carbon Nanostructures. 2014, 22(6), 575–594
5. Monajjemi,M.;Mahdavian, L.;Mollaamin,F. Bull Chem.Soc.Ethiop.2008,22(2), 1-10
6. Monajjemi,M.;Seyed Hosseini, M.;Molaamin,F.Fullerenes Nanotubes and Carbon Nanostructures. 2013, 21, 381–393
7. Mollaamin, F.;Najafpour, J.;Ghadami,s.;Ilkhani,A. R.;Akrami,M. S.;Monajjemi,M. J. Comput. Theor.Nanosci.2014,11(5), 1290-1298
8. Mollaamin,F.;Baei,M.T.; Monajjemi,M..; Zhiani,R.; Honarparvar,B.Russian Journal of Physical Chemistry A. 2008,82 (13), 2354-2361
9. Bhupesh Bishnoi and BahnimanGhosh,2014, Quantum Matter 3, 469-475.
10. SuleCelasun , Rev. Theor. Sci. 1,2013, 319-343
11. Akshay kumar Salimath and Bahniman Ghosh ,Quantum Matter,2014, 3, 72-77.
12. Monajjemi,M.;Seyed Hosseini,M.; Mousavi,M.;Jamali,Z. Fullerenes, Nanotubes, and Carbon Nanostructures. 2014,22, 798–808
13. Mollaamin,F.; Shahani pour,K.;Shahani pour,K.;Ilkhani,A. R.;Sheckari,Z.;Monajjemi,M.Russian Chemical Bulletin. 2012,61(12),2193-2198
14. Monajjemi,M.; Najafpour, J.;Mollaamin,F.;Fullerenes, Nanotubes, and Carbon Nanostructures, 2013, 21: 213–232
15. Monajjemi,M.; Hosseini,SeyedM.;Journal of Computational and Theoretical Nanoscience,2013,10 (10):2473-2477
16. Davide Fiscaletti and Amrit Sorli ,Quantum Matter,2014, 3, 200-214.
17. Monajjemi,M.  ; Mahdavian,L.  ; Mollaamin,F.  ; Honarparvar,B. Fullerenes, Nanotubes and Carbon Nanostructures,2010, 18: 45–55
18. Monajjemi,M.;Faham,R.;Mollaamin ,F.Fullerenes, Nanotubes, and Carbon Nanostructures,2012, 20 (2): 163–169.
19. Bjürn Piglosiewicz, Jan Vogelsang, Slawa Schmidt, Doo Jae Park, Petra Gro ß, and Christoph Lienau ,Quantum Matter,2014 3, 297-306.
20. A. M. Ilyin ,Quantum Matter,2013, 2, 205-208.
21. Monajjemi,M.;Mollaamin ,F.;J. Comput. Theor.Nanosci.2012,9, 2208-2214
22. Medhat Ibrahim and HananElhaes ,Rev. Theor. Sci. 1, 2013,368-376
23. Anurag Srivastava, NileshiSaraf, and A. K. Nagawat ,Quantum Matter,2013,2, 401-407.
24. IIJIMASumio, YUDASAKA Masako, NIHEY Fumiyuki, NEC TECHNICAL JOURNAL, 2,1,2007.
25. S. Iijima, Nature,1991, 354, 56
26. S. Iijima and T. Ichihasi, Nature,1993,363, 603
27. D. S. Bethune, C. H. Kiang, M. S. deVries, G. Gorman, R. Savoy, J. Vazques, andR. Beyers, Nature,1993, 363, 605
28. T. Ramanathan, F. T. Fisher, R. S. Ruoff and L. C. Brinson, Chem mater,2005,  17, 1290
29. Monajjemi,M.;Mollaamin ,F. J. Comput. Theor. Nanosci.2012, 9, 597-601
30. Monajjemi,M.;Robert Wayne, Jrand. Boggs,James E.Chemical Physics ,2014,433,1-11
31. Davide Fiscaletti,Rev. Theor. Sci. 1,(2013),103-144
32. Yahyaei,H.;Monajjemi,M. Fullerenes, Nanotubes, and Carbon Nanostructures. 2014,22(4), 346–361
33. J.-Y. Guo, C.-X.Xu, F.-Y.Sheng, Z.-L.Jin, Z.-L.Shi, J. Dai, and Z.-H. Li Quantum Matter,2013, 2,  181-186.
34. Yahyaei,H.;Monajjemi,M. ;Aghaie,H. ;Zare ,K.Journal of Computational and Theoretical Nanoscience,2013, 10(10), 2332–2341
35. Mollaamin, F.;Monajjemi,M.;Mehrzad,J. Fullerenes, Nanotubes, and Carbon Nanostructures.2014, 22 (8): 738–751
36. Monajjemi, M.; Jafari Azan, M.; Mollaamin, F. Fullerenes Nanotubes and Carbon Nanostructures.2013,21(6).
37. Aleksander Herman ,Rev.Theor. Sci. 2013,1, 3-33.
38. Monajjemi,M.;Sobhanmanesh, A.;Mollaamin ,F. Fullerenes, Nanotubes, and Carbon Nanostructures, 2013, 21(1), 47–63.
39. BurcuTüzün and csakirErkoç ,Quantum Matter,2012; 1, 136-148.
40. Mollaamin,F.;NajafiF.;Khaleghian, M.;KhaliliHadad, B.;Monajjemi , M. Fullerenes, Nanotubes, and Carbon Nanostructures,2011, 19(7): 653–667
41. Gaussian 98, Revision A.7. (1998) Frisch, M. J., Trucks, G.W., Schlegel, H. B., Scuseria, G. E.,Robb, M. A., Cheeseman, J. R., Zakrzewski, V. G., et al. Gaussian, Inc., Pittsburgh PA, USA.
42. B. Ghalandari, M. Monajjemi, and F. Mollaamin, Journal of Computational and Theoretical Nanoscience,2012, 8, 1212–1219.
43. Monajjemi,M.; Afsharnezhad,S.; Jaafari,M.R.; Abdolahi,T.; Nikosade,A.;Monajemi,H.Russian Journal of physical chemistry A, 2007; 2,1956-1963
44. Monajjemi, M.; Aghaie, H.;Naderi, F. Biochemistry (Moscow).2007, 72 (6), 799-804
45. Monajjemi, M. Chemical Physics. 2013,425,29-45
46. Monajjemi,M.;Falahati,M;Mollaamin,F.Ionics. 2013, 19, 155–164
47. Monajjemi, M.; Naderi,F. ;Mollaamin,F. ;Khaleghian,M.J. Mex. Chem. Soc. 2012, 56(2), 207-211
48. Monajjemi,M. ;Kharghanian, L. ;Khaleghian,M.;Chegini,H.Fullerenes, Nanotubes, and Carbon Nanostructures, 2014,22, 709–725
49. Youky Ono, NobuhiroKusuno, Koichi Kusakabe, and Naoshi Suzuki , Japanese Journal of Applied Physics, 2009, 48045001.
50. Monajjemi,M.Struct Chem. 2012, 23, 551-580
51. Monajjemi,M. ;Khaleghian,M. J Clust Sci. 2011, 22673
52. Michael Lipkind ,Rev. Theor. Sci. 1, 2013,145-164.
53. Monajjemi,M. ; Boggs,J.E.J. Phys. Chem A.2013, 117 (2013) 1670
54. E. L. Pankratov and E. A. Bulaeva ,Rev. Theor. Sci.2013,1, 58-82.
55. Monajjemi,M. ; Lee,V. S. ;Khaleghian,M. ;Honarparvar,B. ;Mollaamin,F. J. Phys. Chem C, 2010, 114, 15315
56. Toshiaki Natsukia, Qing-Qing Ni, Morinobu Endo. C A R B ON,2008, 46, 1570 –1573
57. Monajjemi,M.;Chegini, H.; Mollaamin,F.;Farahani,P.Fullerenes, Nanotubes, and Carbon Nanostructures.2011,19 (5), 469–482
58. Mollaamin, F.;Monajjemi, M.Physics and Chemistry of Liquids. 2012, 50 (5) , 596–604
59. Tiana, G.; Sutto, L.; Broglia, R.A.; Physica A: Statistical Mechanics and its Applications,2007,380, 241.
60. Liu, J.Ch.; Monson, P.A. Adsorption,2005, 11, 5.
61. Lee C.,YangW.,Parr G.R.Phys.Rev.B.,1998,37,785.
62. Monajjemi ,M. ; Heshmat ,M. ; Haeri ,H. H.Biochemistry (Moscow).2006, 71, 113-122
63. Ardalan  ,T.; ArdalanP.;Monajjemi,M.Fullerenes, Nanotubes, and Carbon Nanostructures. 2014, 22, 687-708.
64. Monajjemi,M.; Honarparvar, B. ;H. Haeri, H.Heshmat ,M.Russian Journal of physical Chemistry C.2006 80(1),40-44
65. Monajjemi, M.; Mollaamin, F. Journal of Cluster Science.2012, 23(2), 259-272
66. Monajjemi,M.; J Mol Model, 2014,20, 2507.
67. David Young, Cytoclonal Pharmaceutics Inc, Introduction to Computational Chemistry.
68. HyperChem 7.0, Hypecube Inc., Gainesville, FL, USA, 2001.
69. MonajjemiM.; Karachi,N.;Mollaamin,F. Fullerenes, Nanotubes, and Carbon Nanostructures. 2014 ,22(7), 643–662
70. Monajjemi,M.;YamolaH.;Mollaamin, F.Fullerenes, Nanotubes, and Carbon Nanostructures. 2014, 22(6), 595–603
71. Monajjemi ,M.;Noei ,M.;Mollaamin,F.Nucleosides, Nucleotides and Nucleic Acids. 2010, 29, 676–683

---

This work is licensed under a Creative Commons Attribution 4.0 International License.