IR, Raman and Ab-initio Calcualtions of Glycolic Acid

Introduction

Biodegradable polymers can be efficiently utilized for various purposes such as drug delivery, orthopaedic, dental, and tissue engineering^1-7. Such sophisticated applications usually require polymers with narrowly defined material properties. For a polymer to be used in drug delivery system, it is desired that it should degrade in prerequisite manner^8-12. Degradable aliphatic polyesters (bearing ester linkage-CH2-COO-) are having special significance in drug delivery systems as the ester bonds can be cleaved under physiological conditions in absence of proteolytic activity¹³. Among the polyesters, the polymers derived from α -hydroxy acids have found the most extensive use. Poly (α-hydroxy acids) such as poly (glycolic acid) PGA and poly (lactic acid)  having excellent mechanical properties and biological affinity are the most widely studied polymers. However their crystallinity, hydrophobic nature and lack of functional diversity in the back bone have interfered with modulation of their degradation state, mechanical properties and morphology. Studying carboxyl group (COOH) and its interactions are very important in many  areas of science: such as surface science^14,15, electrochemistry^16,17, and biology^18,19. In environment, COOH of humic acid plays a crucial role in  speciation, transport and deposition of metal ions^20-23. It is one of the important  groups leading to the reactivity of humic substances^24-26. Furthermore, trace metals could interact with humic substances as a result of  electrostatic attraction  and/or formulation of a chelate structure to a charged COO group²⁵. COOH of both formic and carboxylic acids possess potentially two proton binding sites namely  OH and C=O groups. Proton bound clusters are known to form hydrogen bounded networks²⁷. Many proton bounded clusters have been investigated experimentally^28,29 as well as through molecular modeling^30,31. In the present work, the infrared, Raman and theoretical calculations of the frequencies for glycolic acid are reported.

Experimental

The FT-IR spectrum was recorded using a Perkin-Elmer FT-IR spectrometer. The spectral resolution was 4 cm^-1. Standard KBr technique with  1 mg sample per 300 mg KBr was used. The FT-Raman spectrum was obtained on a Bruker IFS 66V NIR-FT instrument equipped with a FRA 106 Raman module. A Nd/YAG laser at 1064 nm with an output on 300mw was used as the  exciting source.

Computational Details

Calculations of Glycolic acid are carried out with Gaussian03³² program  using the Hartree-Fock/6-31G* basis set to predict the molecular structure and vibrational wave numbers. Molecular geometry was fully optimized by Berny’s optimization algorithm using redundant internal coordinates. Harmonic vibrational wave numbers are calculated using the analytic second derivatives  to confirm the convergence to minima on the potential surface. The wave number  values computed at the HF level contain known systematic errors due to the negligence of electron correlation³³. We therefore have used the scaling factor  value of 0.8929 for HF/6-31G* basis set. Parameters corresponding to optimized geometry of Glycolic acid (figure 1) is given in table 1. The absence of imaginary  wave numbers on the calculated vibrational spectrum confirms that the structure deduced corresponds to minimum energy.

Results And Discussion

The observed Raman and IR bands with their relative intensities, calculated values and assignments are given in Table 2.

The vibrations of the CH₂ group, the asymmetric stretch υ_asCH₂, symmetric stretch υ_sCH₂, scissoring vibration δCH₂ appears in the region 2925 ± 10, 2855 ± 10, 1463 ± 13 cm^-1 respectively³⁴. The positions of the C-H stretching vibrations are among the most stable in the spectrum. The HF calculation gives υ_asCH₂ at 2936 cm^-1 and υ_sCH₂ at 2895 cm^-1. The weak band observed in the  IR spectrum at 2940 cm^-1 and 2945 cm^-1 in Raman spectrum is assigned as the  asymmetric CH₂ stretching mode. The symmetrical CH₂ group stretching bands is observed at 2914 cm^-1 in the IR spectrum. The CH₂ in-plane deformation band  which comes near 1463 cm^-1 in alkenes³⁵ is lowered about 1440 cm^-1, when  the CH₂ group is next to a double or triple bond. A carbonyl, nitrite or nitro group  each lower the wave number³⁵ of the adjacent CH₂ group to about 1425 cm^-1. In the present case band observed at 1467 cm^-1 in the Raman spectrum and 1482 cm^-1 (HF) are assigned to the scissoring mode of CH₂. Absorption of hydrocarbons  due to CH₂ twisting and wagging vibration, is observed in the 1350-1150 cm^-1 region^35,36. These bands are generally appreciably weaker than those resulting from  CH₂ scissoring vibration. The CH₂ wagging and twisting modes are assigned at  1309 cm^-1 and 1245 cm^-1 theoretically. These bands are observed at 1357 cm^-1, 1264 cm^-1 in the IR spectrum and at 1233 cm^-1 in the Raman spectrum. The rocking  modes³⁴ ρCH₂ is expected in the range 895±85 cm^-1. The bond calculated at  835 cm^-1 is assigned as the rocking mode ρCH₂. The band at 814 cm^-1 in the IR spectrum and 893 cm^-1 in the Raman spectrum are assigned as ρCH₂ modes for the title compound. The torsional modes are seen in the low wave number range³⁴.

Carboxylic acids are best characterized by the OH stretch, the C=O stretch and the OH out-of-plane deformation and even by the C-O stretch and the OH in-plane deformation. The C=O stretching vibration in the spectra of carboxylic  acids³⁴ give rise to a strong band in the region 1725 ± 65 cm^-1. In the vapor state the  monomer absorbs at a wave number 50 cm^-1 higher. In the present case  we have observed at band at 1729 cm^-1 in the IR spectrum and 1714 cm^-1 in the  Raman spectrum. The HF calculation gives the mode at 1709 cm^-1 as υC=O. The C=O in-plane deformation is weakly to moderately active in the region 725±95 cm^-1.  In the present case 619 cm^-1 (HF) and 619 cm^-1 (IR) are assigned as δC=O of carboxylic group. Most carboxylic acids display γC=O in the region 595 ± 85 cm^-1 which is  in the vicinity of that of methyl and ethyl esters. For the title compound  the  band is at 496 cm^-1 in the Raman spectrum, 499 cm^-1 in the IR spectrum and 499 cm^-1 (HF)  is assigned in the γC=O mode. Two bands arising from the C-O stretching and O-H bending appear in the spectra of carboxylic acids near 1320-1210 cm^-1 and 1440-1395 cm^-1 respectively³⁶. Both of these bands involve some interaction between C-O stretching and in-plane C-O-H bending. The υ(C-O)c mode is reported at 1377 cm^-1 for sodium salicylate³⁷ at 1391 cm^-1 for 4-amino salicytic acid³⁸ and at 1375 cm^-1 (IR), 1382 cm^-1 (HF) for 3,5 dinitro salycytic acid³⁹. For the title compound  the band observed at 1125 cm^-1 (HF) and 1116 cm^-1 Raman and 1154 cm^-1 in IR  are assigned as υ(C-O)c mode. The torsional modes are seen in the low wave number  range³⁴. The OH stretching mode is observed at 3600 cm^-1 in the IR spectrum as expected³⁴. In the present case HF calculation gives the δOH mode at 1421 and 1432 cm^-1 (IR) and 1431 cm^-1 in the Raman spectrum.

In the OH band of oxymethyl group the υOH stretching vibration is expected in the region 3300 ±120 cm^-1. In the present case the HF calculation give this value at 3561 cm^-1 and 3600 cm^-1 in the IR spectrum. The OH deformation band is expected in the region³⁴ 1400±40 cm^-1. In OH in-plane deformation the bands is observed at 1218 cm^-1 (HF), 1229 cm^-1 (IR) are assigned as δOH oxymethyl group. The out-of plane deformation is expected at 640±70 cm^-1. In the present case HF calculation gives the mode at 620 cm^-1 and Raman at 683 cm^-1 The stretching vibration υCO is expected at 1045 ± 45 cm^-1. The band at 1083 cm^-1 in  the Raman spectrum, 1086 cm^-1 in the IR spectrum and 1047 cm^-1 (HF) are assigned as υCO modes for the title compound. The δ-C-O and ρ(C=O) O bands are calculated to be at 450 cm^-1 (HF) and at 457 cm^-1 in the IR spectrum. The other modes are also identified and tabulated (Table 2)

For the title compound the bond lengths C₁-O₃=1.2117, C₁-O₂=1.5316, C₅-O₈=1.4139Å  and these values are in assignment with the reported values 1.203, 1.2074Å (C=O), 1.361, 1.3487Å(C-O)^40.41. The ab initio calculation give the O-H bond lengths as O₈-H₉= 0.9541, O₂-H₄=0.9548 Å, where as the reported values are 0.9650, and 0.9533Å^40,41. At C₁ the bond angle are O₂-C₁-O₃=123.3, O₂-C₁-O₅=112.5, O₃-C₁-C₅=124.3° and the deviation from 120˚ shows that the interaction between COOH group and the CH₂ group. C₁-C₅=1.4974, C-H=1.0809Å the C-C and C-H  bond lengths are 1.4974 and 1.0809 Å which are in agreement with the reported values^42,43. The C₅-O₈-H₉ angle is 113.3°, which shows hydrogen bond between O₃ and H₉. The calculated first hyperpolarizability of the title compound is 0.562 × 10^-30 esu and is an attractive object for future studies of nonlinear optics.

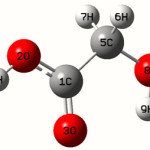Scheme 1 Click here to View scheme

 

Conclusion

The IR and Raman spectrum of Glycolic acid were recorded and analysed. The frequencies are calculated theoretically using Gaussian03 software package. The calculated frequencies are found to be in agreement with the experimental values . The geometrical parameters of the title compound are in agreement with the reported values of similar compounds. The vibrational modes are assigned with the help of reported literature.

Table 1: Optimized geometrical parameters of the title compound

Bond Lengths (A˚)	Bond angles (˚)	Dihedral angles (˚)
C1-O2       1.5316 C1=O3      1.2117 C1-C5       1.4974 O2-H4       0.9548 O8-H9       0.9541 C5-H6       1.0809 C5-H7       1.0809 C5-O8       1.4139 C5-H9       0.9541	A(2,1,3)          123.3 A(2,1,5)          112.5 A(3,1,5)          124.3 A(1,2,4)          114.7 A(1,5,6)          108.9 A(1,5,7)          108.9 A(1,5,8)          111.2 A(6,5,7)          108.0 A(6,5,8)          109.9 A(7,5,8)          109.9 A(5,8,9)          113.3	D(3,1,2,4)                -0.0 D(5,1,2,4)            -180.0 D(2,1,5,6)                58.8 D(2,1,5,7)               -58.8 D(2,1,5,8)             -180.0 D(3,1,5,6)             -121.2 D(3,1,5,7)              121.2 D(3,1,5,8)               -0.01 D(1,5,8,9)                0.03 D(6,5,8,9)              120.7 D(7,5,8,9)             -120.6

Table 2: Infrared, Raman spectral data and calculated wave numbers and band

    assignments  for Glycolic Acid

υ(HF) (cm-1)	IR intensities (KM/Mole)	Raman Activity (Å4/AMU)	υ(IR) (cm-1)	υ(Raman) (cm-1)	Assignments
3562	116.77	87.81	3600	–	υOH
3561	83.98	41.45	–	–	υOH
2936	14.24	70.23	2940	2945	υasCH2
2895	12.96	121.07	2914	–	υsCH2
1709	332.76	4.80	1729	1714	υC=O
1482	15.52	15.18	–	1467	δCH2
1421	7.50	2.94	1432	1431	δOH
1309	118.87	3.44	1357		ωCH2
1245	77.29	2.25	1264	1233	τCH2
1218	0.05	13.33	1229	–	δOH
1125	164.17	3.97	1154	1116	υC-O
1047	242.13	5.02	1086	1083	υCO
1030	3.66	0.15	1004	1083	υC-C
835	45.70	7.99	814	893	ρCH2
620	31.16	5.42	–	683	γOH
619	266.68	1.00	619	–	δC=O
499	23.72	3.92	499	496	γC=O
450	33.38	2.61	457	–	δ-C-O, ρ(C=O)O
266	8.42	0.09	–	250	tCOH
223	132.75	2.00	–	–	tCOOH
24	65.77	0.27	–	–	tCH2

υ-stretching; ω-wagging; δ-in-plane deformation ;γ-out-of-plane deformation;

ρ-rocking ;τ-twisting; t-torsional; subscript: as-

asymmetric; s-symmetric.

References

1. Kulkarni, R.K., Moore, E.G., Hegyeli, A.F., and Leonard, F., J. Biomed. Mat. Res. 5: 169 (1971).
2. Miller, R.A., Brady, J.M., and Cutright, D.E., J. Biomed. Mat. Res. 5: 711 (1977).
3. Based on a lecture presented at the Fourth annual Biomaterial Symposium, ClemsonUniversity, Clemson, South Sarolina, Getter, (1972).
4. Jackanicz,  T.M., Nash, H.A., Wise, D.L.,  and Gregory, J.B., Contraception, 8: 227 (1973)227.
5. Anderson, L.C., Wise, D.L., and Howes, J.F., Contraception, 13: 375 (1976).
6. Schmitt,  E.E., and Epstein, M.A., US patent 3718150, 1971.
7. Schurope, A.D., Wise, D.L., and Howes, J.F., Life Sci. 17: 1877 (1975).
8. Schmitt, E.E., Epstein, M., and Polistina, R.A., US patent 3422871, 1969.
9. Schmitt, E.E., and Polistina, R.A., US patent 3297033, 1967.
10. Frazza, E.J., and Schmitt, E.E,  J. Biomed. Mat. Res. 5: 43 (1971).
11. Kumar, G.S, Kalpagam, V., and Nandi, U.S., Rev. Macromole. Chem. Phys. 22: 225 (1982).
12. Willians, D.F, J. Mat. Sci. 17: 1233 (1982).
13. Woud, D.A, Int. J. Pharm. 7: 11 (1980).
14. Smith, D.A., Wallwork, M.L., Zhang, J., Kirkhan, J., Robinson, C., Marsh, A., and Wong, M., J. Phys. Chem. B,104: 8862 (2000).
15. Kokkoli, E., and Zukoski, C.F., Langmuir, 17: 369 (2001).
16. Boubour, E., and Lennox, R.B., Langmuir,16: 4222 (2000).
17. Sugihara, K., Shimazu, K., and Uosaki,  K., Langmuir, 16: 7101 (2000).
18. Franco, M., Nealy, P.E., Campbell, S., Teixeria, A.I., and Murphy, C.J., J. Biomed. Mat. Res., 52: 261 (2000).
19. Champman, R.G., Ostuni, E., Yan, L., and Whitesides, G.M., Langmuir, 16: 6927 (2000).
20. MacCarthy, P., Aquatic humic substances and their influence in the fate and treatment of pollutants., Edited by Suffect, I.H., and MacCarthy, P., Am. Chem. Soc.Washington, DC, (1989) pp-17-30.
21. Livens, F.R., Environ. Pollut., 70: 183 (1991).
22. Pandey, A.K., Pandey, S.D., and Misrav, V., Ecotoxicol. Environ. Safety, 47: 195  (2000).
23. Tsutsuki, K., and Kuwatsuka, S., Soil Sci. Plant Nutr., 24: 547 (1978).
24. Perdue, E.M., Reuter, J.H., and Ghost, M., Geochim. Cosmochin. Acta, 44: 1841 (1980).
25. Hunns,  F.J.S., Chemistry:Genesis, Composition Reactions., 2^nd editions,  John Wiley and Sons, Inc. New York (1994)
26. Lifsshitz, C., and Louagef, L., J. Phys. Chem., 93: 5633 (1989).
27. Feng, W.Y.,and  Lifshitz, C., J. Phys. Chem., 98: 6075 (1994).
28. Lifshitz, C., and Feng, W.Y., Int. Mass Spectrum Ion Proc. 146: 223 (1995).
29. Hirata, H., and Iwata, S., J. Phys. Chem., 102: 8426 (1998).
30. Colomins, C., Teixido, J., Cemeli, J., Luque, F.J., and Orozo, F.L.,  J. Phys. Chem. B, 102: 2269 (19980).
31. Zhang, R.Q., Wong, N.B., Lee, S.T., Zhu, R.S., and Han, K.L., Chem. Phys Lett., 319: 213 (2000).
32. Frisch, M.J., et al. Gaussian03, Gaussian Inc; Wallingford CT (2004).
33. Foresman, J.B., and Frisch, E., in: Exploring Chemistry With Electronic Structure Methods: A Guide to Using Gaussian, Gaussian Pittsburg, PA (1996).
34. Roeges, N.P.G., A Guide to the complete Interpretation of Infrared Spectra of Organic structure, Wiley, New York (1994).
35. Colthup, N.B., Daly, L.H., and Wiberly, S.E., Introduction to Infrared and Raman spectroscopy,  3^rd ed; Academic press, Boston (1990).
36. Silverstein, R.M., Bassler, G.C., and Morril, T.C., Spectrometric Identification of Organic compounds, 5th. ed; John Wiley and Sons Inc; Singapore (1991).
37. Philip, D.,  John, A., Panicker, C.Y.,  and Varghese, H.T., Spectrochim. Acta A 57: 1561 (2001).
38. Panicker, C.Y., Varghese, H.T., John, A., Philip, D.,  Istvan, K., and Keresztury, G., Spectrochim.  Acta A  58: 281 (2002).
39. Varghese, H.T., Panicker, C.Y., Philip,  D., Chowdhury, J.,  and Ghosh, M.J., J. Raman Spectrosc. 38: 323 (2007).
40. Navarrete, J.T.L., Bencivenni, L., Ramondo, F., Hernandez, V., and Ramirez, F.J., J. Mol.Struct. (Theocom), 330: 261 (1995).
41. Van Alsenoy, C., Kulp, S., Siam, K.,  Klimkowski, V.J., Ewbank, J.D., and Schafer, L., J. Mol. Struct. (Theocom), 181: 169 (1988).
42. Iijima, K., Tanaka, K., and Onuma, S., J. Mol. Struct. 246: 257 (1991).
43. Iijima, K., and Beagley, B., J. Mol. Struct.,  248: 133 (1991).

---

This work is licensed under a Creative Commons Attribution 4.0 International License.